Increasing the accuracy of the vessel’s course orientation
DOI:
https://doi.org/10.15587/2706-5448.2024.298518Keywords:
intelligent transport systems, automatic control, navigational safety, human factor, inertial deviation, course orientation accuracyAbstract
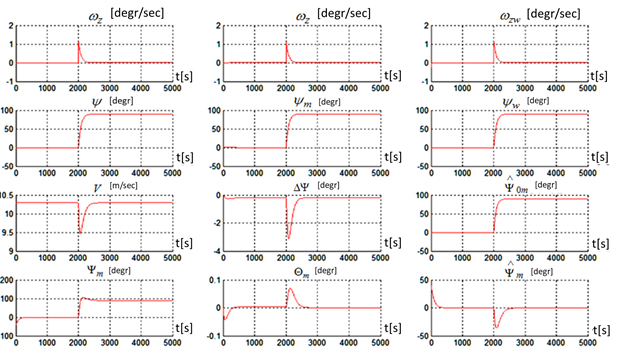
The object of the study is the process of increasing the accuracy of measuring the vessel's course and course orientation by using the mathematical model of the gyrocompass in the on-board computer. On modern vessels, the gyrocompass is the main course measuring device. Its advantages lie in measuring the current course relative to the geographic meridian, its readings are not affected by magnetic anomalies, as was the case when using magnetic compasses, which led to a partial or complete loss of orientation of control objects. At the same time, gyrocompasses also have their drawbacks. The most significant of them is the inertial deviation of the sensitive element caused by the curvature of the Earth's surface, a change in course, acceleration or deceleration of the vessel. With the appearance of the moments of these forces, the axis of the gyrocompass leaves the equilibrium position and begins to make precessional movements. To reduce the inertial deviation, constructive solutions and recommendations to shipmasters regarding the consideration of deviational errors are used. Structural solutions lead to an increase in weight, complexity of the design, a decrease in reliability, and an increase in cost. The recommendations of regulatory documents regarding the consideration of the inertial deviation of the sensitive element of the gyrocompass are difficult to implement in practice, but they can be implemented in the on-board computer of the vessel control system by using a mathematical model of the sensitive element. The paper developed a method of increasing the accuracy of gyrocompass course measurement and the accuracy of course orientation by using an observation device built on the basis of a mathematical model of the gyrocompass in the on-board computer of the course control system. This makes it possible to estimate the useful component of course measurement and deviational errors from changes in speed and course, the curvature of the earth's surface. The useful component, without deviational errors, is used in the vessel's course control channel. The developed method can be used on vessels, provided it is integrated into the existing automated system of the on-board computer to solve the problem of monitoring the components of the gyrocompass measurement.
References
- Nesterenko, O. I., Sapehin, O. M. (2022). Hiroskopichni i navihatsiini prylady. Kyiv: KPI im. Ihoria Sikorskoho, 138.
- Matematychni modeli datchykiv informatsii. Available at: https://er.nau.edu.ua/bitstream/NAU/11005/4/4-%D0%93%D0%BB_3.pdf
- Kulikov, D. O., Kupina, O. A., Loriia, M. H., Tselishchev, O. B. (2022). Use of a mathematical model for optimization of dynamic parameters of ammonia production processes. Visnik of the Volodymyr Dahl East Ukrainian National University, 5 (275), 49–54. doi: https://doi.org/10.33216/1998-7927-2022-275-5-49-54
- Kupina, O. A., Loriia, M. H., Tselishchev, O. B., Homa Akhmed, H. A. (2021). Identification of dynamic characteristics of control objects. Visnik of the Volodymyr Dahl East Ukrainian National University, 6 (270), 129–134. doi: https://doi.org/10.33216/1998-7927-2021-270-6-129-134
- Melashchenko, O. M., Volovyk, B. V. (2012). Analiz tochnosti alhorytmiv intehruvannia kinematychnykh rivnian rukhu tverdoho tila. Available at: https://ela.kpi.ua/bitstream/123456789/7305/1/p_09.pdf
- Plotnikov, P. K., Mikheev, A. V., Naumov, S. G. (2013). Pogreshnosti besplatformennogo girokompasa dlia obektov s neogranichennymi uglami povorotov. Available at: http://optolink.ru/documents/Publications/Our/%D0%9C%D0%9A%D0%98%D0%9D%D0%A1-2013%20%D0%9F%D0%BB%D0%BE%D1%82%D0%BD%D0%B8%D0%BA%D0%BE%D0%B2.pdf
- Vikulin, I. M., Mikhailov, N. S., Mikhailov, S. A. (2020). Electronic compass for unmanned ships. Shipping & Navigation, 30 (1), 42–50. doi: https://doi.org/10.31653/2306-5761.30.2020.42-50
- Alekseychuk, M. S., Chapchay, P. A., Avramenko, E. A. (2020). Decline of lateral displacement of ship after a maneuver by the input of additional amendment of the course. Shipping & Navigation, 30 (1), 8–15. doi: https://doi.org/10.31653/2306-5761.30.2020.08-15
- Ivanov, S., Oliinyk, P., Virchenko, G. (2022). Improving an analytical gyroscope azimuth mode to compensate for the ballistic deviation of a marine gyrocompass. Eastern-European Journal of Enterprise Technologies, 3 (5 (117)), 36–46. doi: https://doi.org/10.15587/1729-4061.2022.259024
- Bogolyubov, V., Bakhtieva, L. (2021). Compensation Gyrocompass Based on MEMS. Journal of Physics: Conference Series, 2096 (1), 012203. doi: https://doi.org/10.1088/1742-6596/2096/1/012203
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2024 Mykola Khlopenko, Igor Gritsuk, Oleksandr Sharko, Eduard Appazov

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.








