Contribution to micromechanical modeling of the shear wave propagation in a sand deposit

Authors

DOI:

https://doi.org/10.15587/2706-5448.2024.301709

Keywords:

micromechanical model, sand deposit, discrete element method, shear wave propagation

Abstract

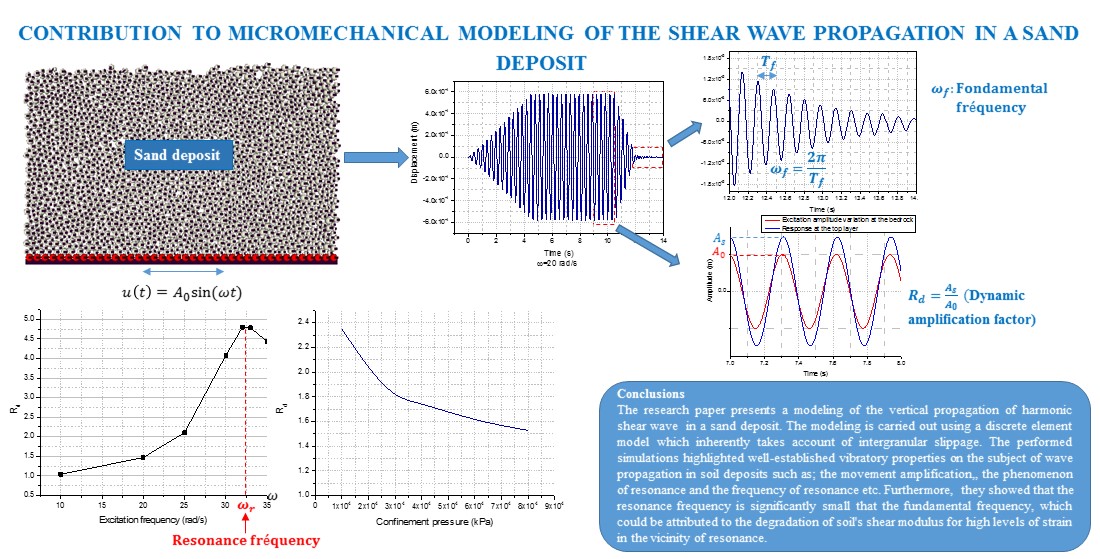
The object of study is the vertical wave propagation in a sand deposit. This paper is aimed at analyzing the vertical wave propagation in a sand deposit through micromechanical modeling that inherently takes account of intergranular slips during deformation. Such a problem, which is part of the general framework of wave propagation in the soil, has long been analyzed using continuum models based on approximate behavior laws. For this purpose, a 2D Discrete Element Method (DEM) model is developed. The DEM model is based on molecular dynamics with the use of circular shaped elements. The intergranular normal forces at contacts are calculated through a linear viscoelastic law while the tangential forces are calculated through a perfectly plastic viscoelastic model. A model of rolling friction is incorporated in order to account for the damping of the grains rolling motion. Different boundary conditions of the profile have been implemented; a bedrock at the base, a free surface at the top and periodic boundaries in the horizontal direction. The sand deposit is subjected to a harmonic excitation at the base. Using this model, the fundamental and resonance frequencies of the deposit are first determined. The former is determined from the low-amplitude free vibration and the latter by performing a variable-frequency excitation test. It is noted that there is a significant gap between the two frequencies, this gap could be attributed to the degradation of the soil shear modulus in the vicinity of the resonance. Such degradation is well proven in classical soil dynamics. The effects of deposit height and confinement on resonance frequency and free-surface dynamic amplification factor are then investigated. The obtained results highlighted that the resonance frequency is inversely proportional to the deposit’s thickness whereas the dynamic amplification factor Rd increases with the deposit’s thickness. In the other hand, when the confinement increases the deposit becomes stiffer, which results in reducing the amplification. Such result is in accordance with theoretical knowledge which states that the most rigid profiles such as rocks do not amplify seismic movement.

Author Biographies

Said Derbane, University of 20 August 1955 Skikda

Postgraduate Student

Department of Civil Engineering

LMGHU Laboratory

Mouloud Mansouri, Ferhat Abbas University of Setif

Associate Professor

Civil Engineering Research Laboratory of Setif (LRGCS)

Department of Civil Engineering

Salah Messast, University of 20 August 1955 Skikda

Professor

Department of Civil Engineering

LMGHU Laboratory

References

  1. Jiang, M., Kamura, A., Kazama, M. (2022). Numerical study on liquefaction characteristics of granular materials under Rayleigh-wave strain conditions using 3D DEM. Soils and Foundations, 62 (4), 101176. doi: https://doi.org/10.1016/j.sandf.2022.101176
  2. Cui, J., Men, F., Wan, X. (2004). Soil liquefaction induced by Rayleigh wave. 13th World Conference on Earthquake Engineering.
  3. Nakase, H., Takeda, T., Oda, M. (1999). A simulation study on liquefaction using DEM. Proceedings of the 2nd International Conference on Earthquake Geotechnical Engineering, 637–642.
  4. Guo, Y., Zhao, C., Markine, V., Jing, G., Zhai, W. (2020). Calibration for discrete element modelling of railway ballast: A review. Transportation Geotechnics, 23. doi: https://doi.org/10.1016/j.trgeo.2020.100341
  5. Kumar, N., Suhr, B., Marschnig, S., Dietmaier, P., Marte, C., Six, K. (2019). Micromechanical investigation of railway ballast behavior under cyclic loading in a box test using DEM: Effects of elastic layers and ballast types. Granular Matter, 21, 106. doi: https://doi.org/10.1007/s10035-019-0956-9
  6. Zamani, N., El Shamy, U. (2011). Analysis of wave propagation in dry granular soils using DEM simulations. Acta Geotechnica, 6 (3), 167–182. doi: https://doi.org/10.1007/s11440-011-0142-7
  7. Sadd, M. H., Adhikari, G., Cardoso, F. (2000). DEM simulation of wave propagation in granular materials. Powder Technology, 109 Ž2000, 222–233. doi: https://doi.org/10.1016/s0032-5910(99)00238-7
  8. Sakamura, Y., Komaki, H. (2011). Numerical simulations of shock-induced load transfer processes in granular media using the discrete element method. Shock Waves, 22 (1), 57–68. doi: https://doi.org/10.1007/s00193-011-0347-6
  9. Ning, Z., Khoubani, A., Evans, T. M. (2015). Shear wave propagation in granular assemblies. Computers and Geotechnics, 69, 615–626. doi: https://doi.org/10.1016/j.compgeo.2015.07.004
  10. Tang, X., Yang, J. (2021). Wave propagation in granular material: What is the role of particle shape? Journal of the Mechanics and Physics of Solids, 157, 104605. doi: https://doi.org/10.1016/j.jmps.2021.104605
  11. Peters, J. F., Muthuswamy, M., Wibowo, J., Tordesillas, A. (2005). Characterization of force chains in granular material. Physical Review E, 72 (4). doi: https://doi.org/10.1103/physreve.72.041307
  12. Fu, L., Zhou, S., Guo, P., Wang, S., Luo, Z. (2019). Induced force chain anisotropy of cohesionless granular materials during biaxial compression. Granular Matter, 21 (3). doi: https://doi.org/10.1007/s10035-019-0899-1
  13. Fu, L., Zhou, S., Zheng, Y., Zhuang, L. (2023). Characterizing dynamic load propagation in cohesionless granular packing using force chain. Particuology, 81, 135–148. doi: https://doi.org/10.1016/j.partic.2023.01.007
  14. Campbell, C. S. (2003). A problem related to the stability of force chains. Granular Matter, 5 (3), 129–134. doi: https://doi.org/10.1007/s10035-003-0138-6
  15. Pöschel, T., Schwager, T. (2005). Computational Granular Dynamics – Models and Algorithms. Berlin, Heidelberg: Springer-Vrlag.
  16. Mansouri, M., El Youssoufi, M. S., Nicot, F. (2016). Numerical simulation of the quicksand phenomenon by a 3D coupled Discrete Element – Lattice Boltzmann hydromechanical model. International Journal for Numerical and Analytical Methods in Geomechanics, 41 (3), 338–358. doi: https://doi.org/10.1002/nag.2556
  17. Richefeu, V. (2005). Approche par éléments discrets 3D du comportement de matériaux granulaires cohésifs faiblement contraints. Thèse Université Montpellier II – Sciences et Techniques du Languedoc.
  18. Cundall, P. A., Strack, O. D. L. (1979) A discrete numerical model for granular assemblies. Géotechnique, 29 (1), 47–65. doi: https://doi.org/10.1680/geot.1979.29.1.47
  19. Delenne, J., El Youssoufi, M. S., Cherblanc, F., Bénet, J. (2004). Mechanical behaviour and failure of cohesive granular materials. International Journal for Numerical and Analytical Methods in Geomechanics, 28 (15), 1577–1594. doi: https://doi.org/10.1002/nag.401
  20. Semblat, J. F., Luong, M. P. (1998). Wave propagation through soils in centrifuge testing. Journal of Earthquake Engineering, 2 (10), 147–171. doi: https://doi.org/10.1080/13632469809350317
  21. Verruijt, A. (2009). An introduction to soil dynamics (Vol. 24). Springer Science & Business Media. doi: https://doi.org/10.1007/978-90-481-3441-0
  22. Acton, J. R., Squire, P. T., (1985) Solving Equations with Physical Understanding. Bristol: Adam Hilger Ltd., 219.
Contribution to micromechanical modeling of the shear wave propagation in a sand deposit

Downloads

Published

2024-06-29

How to Cite

Derbane, S., Mansouri, M., & Messast, S. (2024). Contribution to micromechanical modeling of the shear wave propagation in a sand deposit. Technology Audit and Production Reserves, 3(1(77), 10–18. https://doi.org/10.15587/2706-5448.2024.301709

Issue

Vol. 3 No. 1(77) (2024): Industrial and technology systems

Section

Mechanics

License

Copyright (c) 2024 Said Derbane, Mouloud Mansouri, Salah Messast

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.