Higher order dispersions effect on high-order soliton interactions
DOI:
https://doi.org/10.15587/2706-5448.2023.277346Keywords:
higher order solitons, soliton fission, dispersions, nonlinearity, optical fiber, supercontinuumAbstract
The object of the research is deleting the interaction of the higher order soliton interaction by introducing the third and fourth order dispersions inside an optical fiber. The results are obtained by the simulation of the nonlinear Schrödinger equation, which models the propagation of solitons in the optical fiber using the method of Fast Fourier Transform.
The interaction of two higher order solitons due to the attraction of their electric field can lead to losing the solitons' properties. Hence, this can prevent the use of solitons in high-bit-rate optical fiber communication systems because it increases the bit error rate, significantly limiting the potential of the communication system. To resolve this problem, we should diminish the bit rate error by avoiding the interaction of the co-propagative solitons when they are too close.
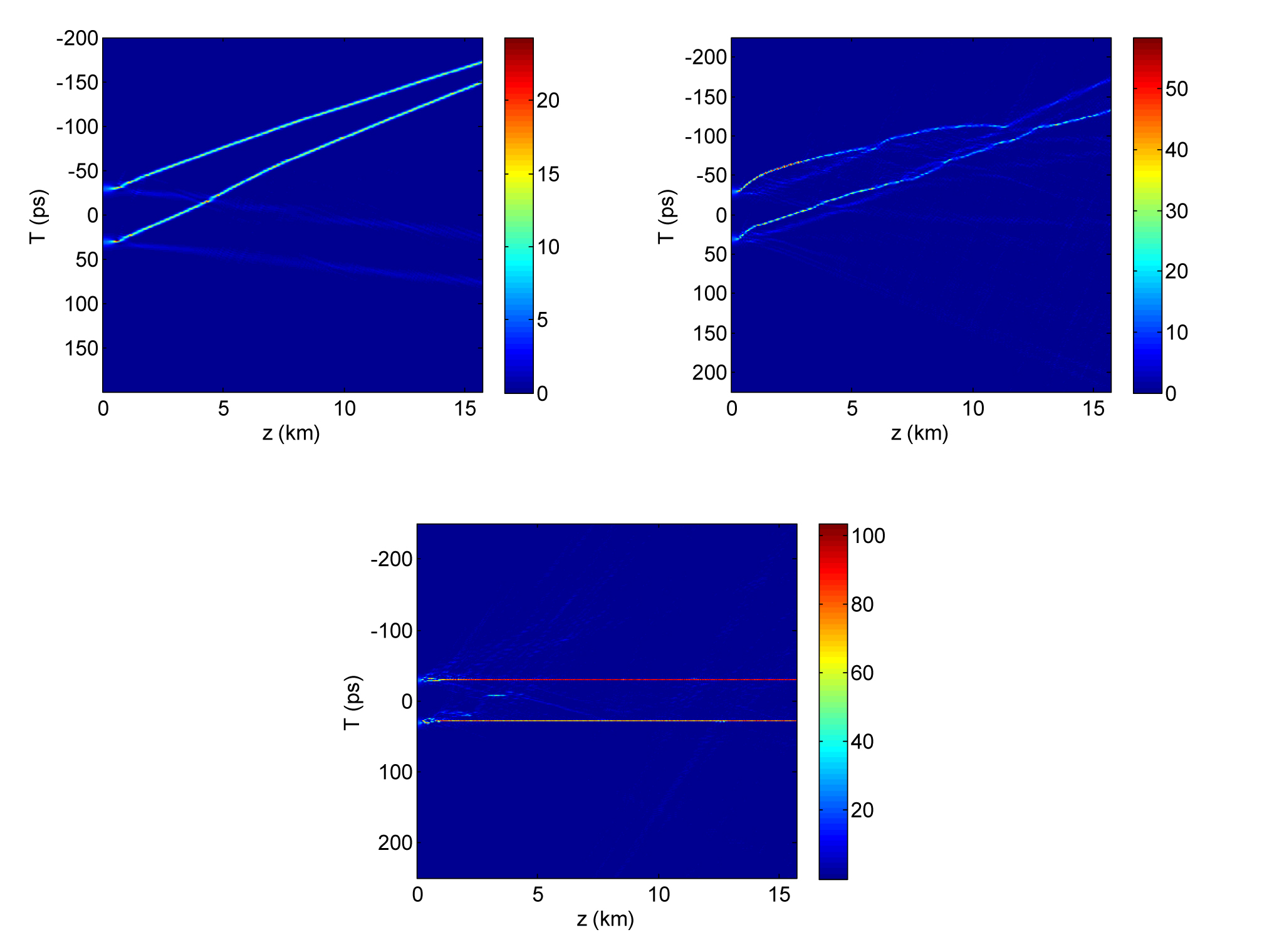
It is well known that, during higher order soliton propagation in the presence of the third order dispersion, the irregular shape of the higher order soliton disappears, and a splitting towards its fundamental constituents occurs after a considerable propagation. As for the fourth order, dispersion gives rise to two dispersive wave sidebands on the red or blue side. Our results reveal that bringing two higher order solitons together in the presence of the fourth order dispersion, a series of interactions between the components generated after their fission is obtained. In the third-order distribution, besides the fourth-order diffusion, the rare form and the supercontinuum generated by the fission of the higher-order solitons disappear, and we get two fundamental solitons propagating in parallel with a temporal shift and some inconsiderable dispersive waves. The most important aspect is that both higher-order dispersions are able to suppress the interactions of higher-order solitons thanks to the time shift induced by the third-order distribution and the intermittent compression caused by the fourth-order scattering. These results can be obtained in practice inside the dispersion-engineered photonic crystal waveguide (PhC-wg), which allows for manipulating the high order dispersion.
References
- Khan, K. R., Mahmood, M. F., Biswas, A. (2014). Coherent Super Continuum Generation in Photonic Crystal Fibers at Visible and Near Infrared Wavelengths. IEEE Journal of Selected Topics in Quantum Electronics, 20 (5), 573–581. doi: https://doi.org/10.1109/jstqe.2014.2302353
- Hernandez-Garcia, J. C., Estudillo-Ayala, J. M., Mata-Chavez, R. I., Pottiez, O., Rojas-Laguna, R., Alvarado-Mendez, E. (2013). Experimental study on a broad and flat supercontinuum spectrum generated through a system of two PCFs. Laser Physics Letters, 10 (7), 075101. doi: https://doi.org/10.1088/1612-2011/10/7/075101
- Dudley, J. M., Genty, G., Coen, S. (2006). Supercontinuum generation in photonic crystal fiber. Reviews of Modern Physics, 78 (4), 1135–1184. doi: https://doi.org/10.1103/revmodphys.78.1135
- Smirnov, S. V., Ania-Castanon, J. D., Ellingham, T. J., Kobtsev, S. M., Kukarin, S., Turitsyn, S. K. (2006). Optical spectral broadening and supercontinuum generation in telecom applications. Optical Fiber Technology, 12 (2), 122–147. doi: https://doi.org/10.1016/j.yofte.2005.07.004
- Lü, X., Zhu, H.-W., Meng, X.-H., Yang, Z.-C., Tian, B. (2007). Soliton solutions and a Bäcklund transformation for a generalized nonlinear Schrödinger equation with variable coefficients from optical fiber communications. Journal of Mathematical Analysis and Applications, 336 (2), 1305–1315. doi: https://doi.org/10.1016/j.jmaa.2007.03.017
- Meng, X.-H., Zhang, C.-Y., Li, J., Xu, T., Zhu, H.-W., Tian, B. (2007). Analytic Multi-Solitonic Solutions of Variable-Coefficient Higher-Order Nonlinear Schrödinger Models by Modified Bilinear Method with Symbolic Computation. Zeitschrift Für Naturforschung A, 62 (1-2), 13–20. doi: https://doi.org/10.1515/zna-2007-1-203
- Yu, T., Golovchenko, E. A., Pilipetskii, A. N., Menyuk, C. R. (1997). Dispersion-managed soliton interactions in optical fibers. Optics Letters, 22 (11), 793–795. doi: https://doi.org/10.1364/ol.22.000793
- Herrmann, J., Griebner, U., Zhavoronkov, N., Husakou, A., Nickel, D., Knight, J. C. et al. (2002). Experimental Evidence for Supercontinuum Generation by Fission of Higher-Order Solitons in Photonic Fibers. Physical Review Letters, 88 (17). doi: https://doi.org/10.1103/physrevlett.88.173901
- Wai, P. K. A., Chen, H. H., Lee, Y. C. (1990). Radiations by «solitons» at the zero group-dispersion wavelength of single-mode optical fibers. Physical Review A, 41 (1), 426–439. doi: https://doi.org/10.1103/physreva.41.426
- Elgin, J. N. (1993). Perturbations of optical solitons. Physical Review A, 47 (5), 4331–4341. doi: https://doi.org/10.1103/physreva.47.4331
- Karpman, V. I. (1993). Radiation by solitons due to higher-order dispersion. Physical Review E, 47 (3), 2073–2082. doi: https://doi.org/10.1103/physreve.47.2073
- Akhmediev, N., Karlsson, M. (1995). Cherenkov radiation emitted by solitons in optical fibers. Physical Review A, 51 (3), 2602–2607. doi: https://doi.org/10.1103/physreva.51.2602
- Roy, S., Bhadra, S. K., Agrawal, G. P. (2009). Dispersive waves emitted by solitons perturbed by third-order dispersion inside optical fibers. Physical Review A, 79 (2). doi: https://doi.org/10.1103/physreva.79.023824
- Wai, P. K. A., Menyuk, C. R., Lee, Y. C., Chen, H. H. (1986). Nonlinear pulse propagation in the neighborhood of the zero-dispersion wavelength of monomode optical fibers. Optics Letters, 11 (7), 464. doi: https://doi.org/10.1364/ol.11.000464
- Biswas, A. (2004). Stochastic perturbation of optical solitons in Schrödinger–Hirota equation. Optics Communications, 239 (4-6), 461–466. doi: https://doi.org/10.1016/j.optcom.2004.06.047
- Biswas, A., Jawad, A. J. M., Manrakhan, W. N., Sarma, A. K., Khan, K. R. (2012). Optical solitons and complexitons of the Schrödinger–Hirota equation. Optics & Laser Technology, 44 (7), 2265–2269. doi: https://doi.org/10.1016/j.optlastec.2012.02.028
- Kohl, R., Milovic, D., Zerrad, E., Biswas, A. (2009). Soliton perturbation theory for dispersion-managed optical fibers. Journal of Nonlinear Optical Physics & Materials, 18 (2), 227–270. doi: https://doi.org/10.1142/s0218863509004592
- Topkara, E., Milovic, D., Sarma, A. K., Zerrad, E., Biswas, A. (2010). Optical soliton perturbation with full nonlinearity in non-Kerr law media. Journal of Optical and Fiber Communications Research, 7 (1-4), 43–59. doi: https://doi.org/10.1007/s10297-010-9007-3
- Biswas, A., Topkara, E., Johnson, S., Zerrad, E., Konar, S. (2011). Quasi-stationary optical solitons in non-kerr law media with full nonlinearity. Journal of nonlinear optical physics & materials, 20 (3), 309–325. doi: https://doi.org/10.1142/s0218863511006108
- Biswas, A., Milovic, D., Savescu, M., Mahmood, M. F., Khan, K. R., Kohl, R. (2012). Optical soliton perturbation in nanofibers with improved nonlinear Schrodinger’s equation by semi-inverse variational principle. Journal of Nonlinear Optical Physics & Materials, 12 (4). doi: https://doi.org/10.1142/s0218863512500543
- Biswas, A., Milovic, D., Girgis, L. (2013). Quasi-stationary optical Gaussons. Optik – International Journal for Light and Electron Optics, 124 (17), 2959–2962. doi: https://doi.org/10.1016/j.ijleo.2012.09.055
- Xu, Y., Jovanoski, Z., Bouasla, A., Triki, H., Moraru, L., Biswas, A. (2013). Optical solitons in multi-dimensions with spatio-temporal dispersion and non-kerr law nonlinearity. Journal of Nonlinear Optical Physics & Materials, 22 (3), 1350035. doi: https://doi.org/10.1142/s0218863513500355
- Biswas, A., Khan, K., Rahman, A., Yildirim, A., Hayat, T., Aldossary, O. M. (2012). Bright and dark optical solitons in birefringent fibers with Hamiltonian perturbations and Kerr law nonlinearity. The Journal of Optoelectronics and Advanced Materials, 14 (7-8), 571–576.
- Bhrawy, A. H., Alshaery, A. A., Hilal, E. M., Manrakhan, W. N., Savescu, M., Biswas, A. (2014). Dispersive optical solitons with Schrödinger–Hirota equation. Journal of Nonlinear Optical Physics & Materials, 23 (1), 1450014. doi: https://doi.org/10.1142/s0218863514500143
- Wang, J., Wang, S., Chu, X., Sun, M. (2013). Numerical Study on Optical Solitons Transmission System with 40 Gbit/s in the Photonic Crystal Fiber. Optics and Photonics Journal, 3 (2), 141–146. doi: https://doi.org/10.4236/opj.2013.32023
- Elgin, J. N., Brabec, T., Kelly, S. M. J. (1995). A perturbative theory of soliton propagation in the presence of third order dispersion. Optics Communications, 114 (3-4), 321–328. doi: https://doi.org/10.1016/0030-4018(94)00602-q
- Biswas, A., Milovic, D. (2009). Optical Solitons with Fourth Order Dispersion and Dual-power Law Nonlinearity. International Journal of Nonlinear Science, 7 (4), 443–447.
- Elshater, M. E. M., Zayed, E. M. E., Al-Nowehy, A-G. (2016). Solitons and other solutions to nonlinear Schrödinger equation with fourth-order dispersion and dual power law nonlinearity using several different techniques. Optik, International Journal for Light and Electron Optics.
- Khelil, K., Saouchi, K., Bahloul, D. (2020). Effect of fourth order dispersion on solitonic interactions. Ukranian Journal of Physics, 4.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2023 Khadidja Khelil, Azzeddine Dekhane, Aissa Benselhoub, Stefano Bellucci

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.








