Research of orthotropic composites failure taking into account their structural stochasticity
DOI:
https://doi.org/10.15587/2706-5448.2023.278121Keywords:
orthotropic composite material, reliability, structure stochasticity, failure loading, distribution function, failure probability, plateAbstract
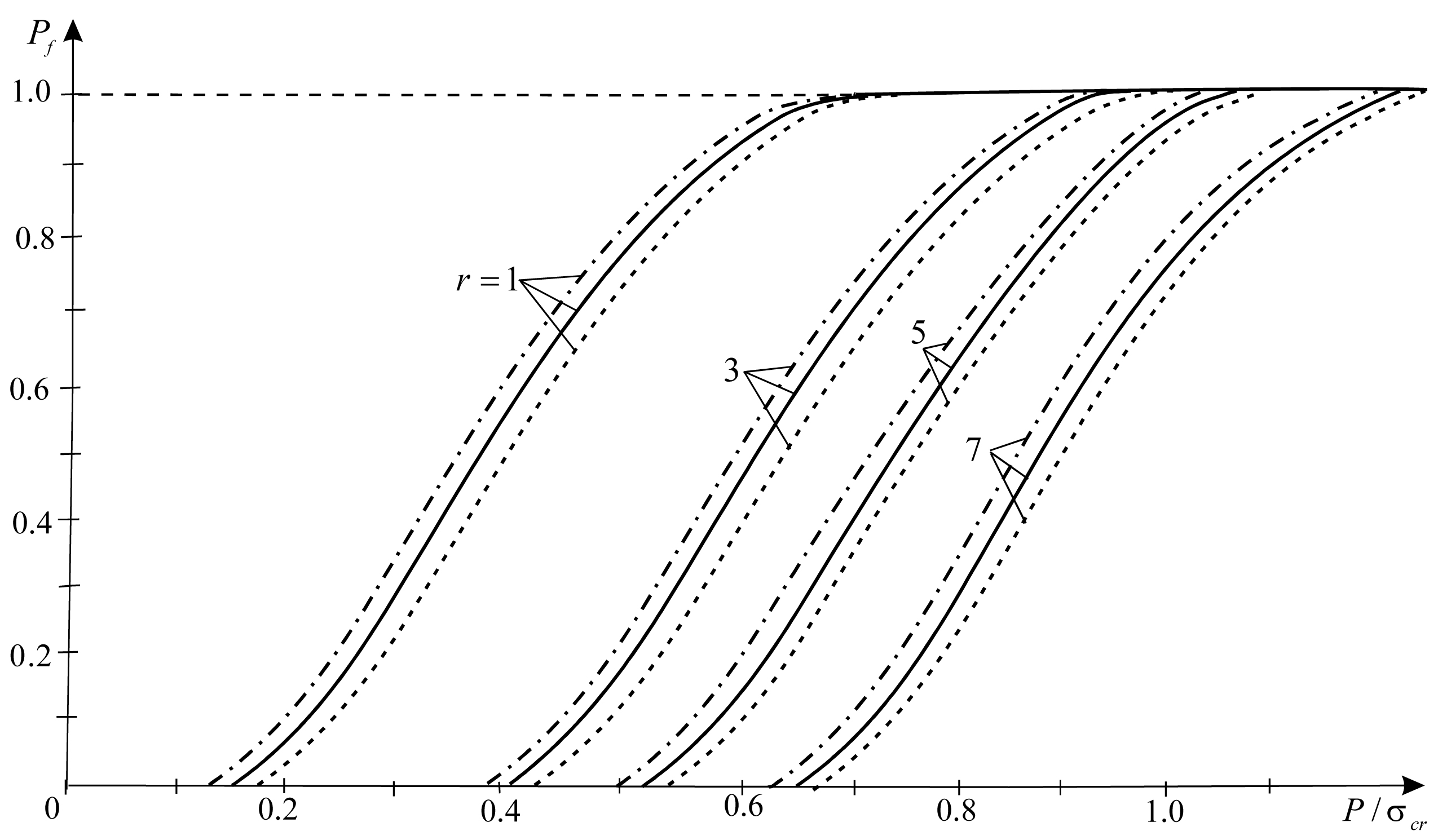
The object of the study is the construction of the reliability assessing algorithm for the orthotropic composite plate, taking into account the stochasticity of its structure under the conditions of plane deformation. The plate consists of a matrix and reinforcement elements. The main orthotropic directions of the material coincide with the directions of the loading. The conducted studies are based on the failure criteria expressed through the components of macro stresses. The hypothesis of the weakest link is used, which for the case of the statistical theory of strength sounds like this: the ultimate (failure) loading for an orthotropic composite plate is equal to the ultimate loading for its weakest element. Defects-cracks are characterized by independent random variables – the half-length and the orientation angle between the defect line and the axis of orthotropy with a higher modulus of elasticity. The proposed model of orthotropic composite material corresponds to known experimental studies epoxy phenolic fiberglass on the cord glass fiber. The distribution probabilities density of defect orientation takes into account their predominant orientation in the direction of reinforcement. On the basis of the obtained composite failure loading integral probability distribution function, the construction and study of the dependence of the plate failure probability diagrams for different number of cracks, structural inhomogeneity and type of applied loading was carried out.
Complex application of the composite materials fracture mechanics deterministic solution and the methods of probability theory and mathematical statistics allows for a more adequate assessment of the composite materials reliability, taking into account the stochasticity of their structure.
The main content of this work is the construction and analysis of dependence of stochastically defective reinforced composite materials failure probability diagrams.
The obtained results make it possible to evaluate the reliability of orthotropic stochastically defective materials under conditions of plane deformation.
The algorithm of a compatible combination of defectiveness and randomness of the orthotropic composite material structure makes it possible to qualitatively investigate its failure under various types of applied loading.
References
- Onkar, A. K., Upadhyay, C. S., Yadav, D. (2007). Probabilistic failure of laminated composite plates using the stochastic finite element method. Composite Structures, 77 (1), 79–91. doi: https://doi.org/10.1016/j.compstruct.2005.06.006
- Sakata, S., Ashida, F., Enya, K. (2012). A Microscopic failure probability analysis of a unidirectional fiber reinforced composite material via a multiscale stochastic stress analysis for a microscopic random variation of an elastic property. Computational Materials Science, 62, 35–46. doi: https://doi.org/10.1016/j.commatsci.2012.05.008
- Gadade, A. M., Lal, A., Singh, B. (2016). Stochastic progressive failure analysis of laminated composite plates using Puck's failure criteria. Mechanics of Advanced Materials and Structures, 739–757. doi: https://doi.org/10.1080/15376494.2015.1029163
- Saberi, S., Abdollahi, A., Inam, F. (2021). Reliability analysis of bistable composite laminates. AIMS Materials Science, 8 (1), 29–41. doi: https://doi.org/10.3934/matersci.2021003
- Xu, J.-H., Zhou, G.-D., Zhu, T.-Y. (2021). Fatigue Reliability Assessment for Orthotropic Steel Bridge Decks Considering Load Sequence Effects. Frontiers in Materials, 8. doi: https://doi.org/10.3389/fmats.2021.678855
- Magar, A., Lal, A. (2021). Progressive failure analysis of laminated plate containing elliptical cutout. International Journal of Structural Integrity, 12 (4), 569–588. doi: https://doi.org/10.1108/ijsi-10-2020-0092
- Baitsar, R., Kvit, R. (2018). Method of the reliability calculation of orthotropic composite materials with random defects. ScienceRise, 10 (51), 28–32. doi: https://doi.org/10.15587/2313-8416.2018.146636
- Serensen, S. V., Zaitcev, G. P. (1982). Nesushchaia sposobnost tonkostennykh konstruktcii iz armirovannykh plastikov s defektami. Kyiv: Naukova dumka, 295.
- Vitvitckii, P. M., Popina, S. Iu. (1980). Prochnost i kriterii khrupkogo razrusheniia stokhasticheski defektnykh tel. Kyiv: Naukova dumka, 186.
- Deliavsky, M., Kvit, R. (1992). Macro-stress distribution near crack-like defects in anisotropic micro-inhomogeneous body under flat deformation and longitudinal displacement. Physicochemical Mechanics of Materials, 2, 50–54.
- Koroliuk, V. S., Portenko, N. I., Skorokhod, A. V., Turbin, A. F. (1985). Spravochnik po teorii veroiatnostei i matematicheskoi statistike. Moscow: Nauka, 640.
- Cheng, A. S., Laird, C. (1981). Fatigue life behaviour of copper single crystals. Part II: Model for crack nucleation in persistent slip bands. Fatigue & Fracture of Engineering Materials & Structures, 4, 343–353. doi: https://doi.org/10.1111/j.1460-2695.1981.tb01131.x
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2023 Roman Kvit

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.








