Finding the optimal solution in pharmaceutical technological research using statistical methods with quantitative factors
DOI:
https://doi.org/10.15587/2519-4852.2024.313819Keywords:
statistical method, regression analysis, multiple-criterion optimization, optimization criterion, pharmacy-technological indicatorAbstract
The aim. Developing a methodological approach to determining the optimal solution using statistical methods in pharmaceutical and technological research with quantitative factors on the example of establishing the optimal content of filler in a solid dispersion in the manufacture of granules.
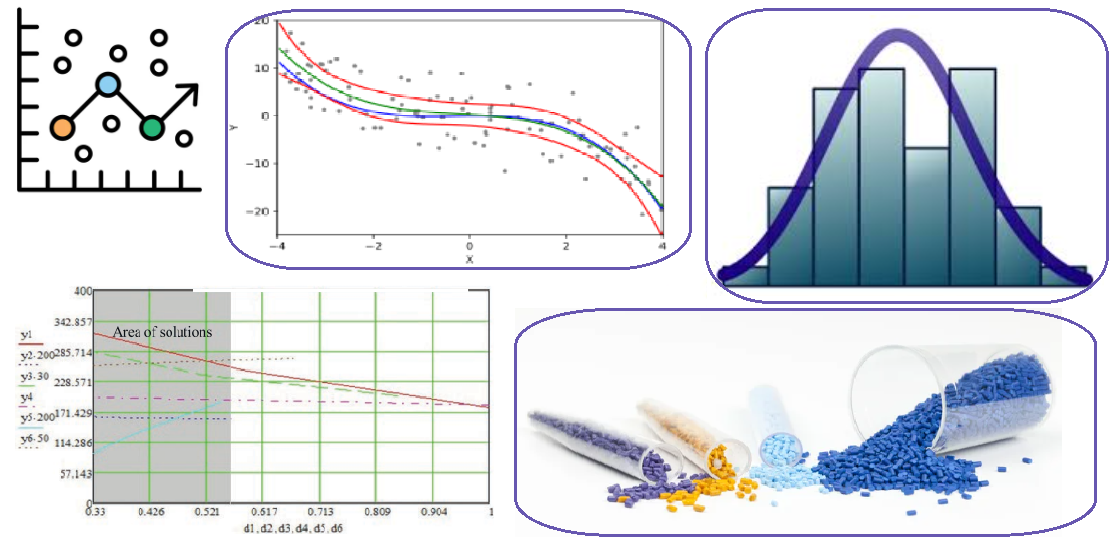
Materials and methods. The methodology for finding an optimal solution is shown in the example of the development of granules containing a solid dispersion of quercetin with polyethylene oxide 6000. To determine the optimal amount of filler, the method of mathematical modelling was applied using statistical analysis and multiple criterion optimization. Software tools such as MS Excel and Mathcad 14 spreadsheets were used to process the experimental data and perform calculations.
Results. The problem is formalized in a form suitable for solving by experimental and statistical methods. The issues of constructing mathematical models in the form of regression equations characterizing the effect of the quantitative content of the filler on several pharmacy-technological parameters of granules based on experimental data are considered. Attention is focused on the peculiarities of using static methods for processing experimental data in pharmaceutical research, namely, on establishing the structure of regression equations and assessing their adequacy. The criterion of multi-criteria optimization is proposed, which is formed based on the obtained regression equations, considering the restrictions imposed on each individual criterion.
Conclusions. A methodological approach to determining the optimal solution in pharmaceutical technological research with quantitative factors using experimental and statistical methods and the theory of multi-criteria optimization has been tested on the example of determining the optimal content of filler in a solid dispersion in the manufacture of granules. The optimal ratio of filler to solid dispersion was determined, which ensures the maximum approximation of all studied pharmacy-technological parameters of granules to their optimal values. None of the indicators exceeds the limits set by the researcher and has the minimum possible deterioration relative to the individually determined optimum
References
- Chatterjee, S., Moore, C. M. V., Nasr, M. M. (2017). Overview of the Role of Mathematical Models in Implementation of Quality by Design Paradigm for Drug Development and Manufacture. Food and Drug Administration Papers. 23. http://digitalcommons.unl.edu/usfda/23
- Piri, Mohsen. (2023). Review of Regression Algorithms. Available at: https://www.researchgate.net/publication/373514743_Review_of_Regression_Algorithms
- Lapach, S. N., Pasichnyk, M. F., Chubenko, A. V. (1999). Statystychni metody v farmakolohii i marketynhu farmatsevtychnoho rynku. Kyiv, 312.
- Frasser, C. (2006). Mathematical methods of experimental data processing and analysis (Or Methods of Curve Fitting). Available at: https://www.researchgate.net/publication/319256581
- Mahbobi, M., Tiemann, T. K. (2015). Introductory Business Statistics with Interactive Spreadsheets. Victoria: BCcampus. Available at: https://opentextbc.ca/introductorybusinessstatistics/
- Henderson, H. V., Velleman, P. F. (1981). Building Multiple Regression Models Interactively. Biometrics, 37 (2), 391–411. https://doi.org/10.2307/2530428
- Odu, G. O. (2013). Review of Multi-criteria Optimization Methods – Theory and Applications. IOSR Journal of Engineering, 3 (10), 1–14. https://doi.org/10.9790/3021-031020114
- Hroshovyi, T. A., Martseniuk, V. P., Kucherenko, L. Y., Vronska, L. V., Hyrieieva, S. M. (2008). Matematychne planuvannia eksperymentu pry provedenyy naukovykh doslidzhen v farmatsii. Ternopil: Ukrmedknyha, 377
- Tanavalee, C., Luksanapruksa, P., Singhatanadgige, W. (2016). Limitations of Using Microsoft Excel Version 2016 (MS Excel 2016) for Statistical Analysis for Medical Research. Clinical Spine Surgery: A Spine Publication, 29 (5), 203–204. https://doi.org/10.1097/bsd.0000000000000382
- Herzog, M. H., Francis, G., Clarke, A. (2019). Understanding Statistics and Experimental Design. Springer Cham, 142. https://doi.org/10.1007/978-3-030-03499-3
- Derzhavna Farmakopeia Ukrainy. Vol. 1 (2015). Kharkiv: Derzhavne pidpryiemstvo «Ukrainskyi naukovyi farmakopeinyi tsentr yakosti likarskykh zasobiv», 1128.
- Divisi, D., Di Leonardo, G., Zaccagna, G., Crisci, R. (2017). Basic statistics with Microsoft Excel: a review. Journal of Thoracic Disease, 9 (6), 1734–1740. https://doi.org/10.21037/jtd.2017.05.81
- Kundrat, A. M., Kundrat, M. M. (2014). Naukovo-tekhnichni obchyslennia zasobamy Mathcad ta MS Excel. Rivne: NUVHP, 252.
- Kovalevska, I., Ruban, O., Kutova, O., Levachkova, J. (2021). Optimization of the composition of solid dispersion of quercetin. Current Issues in Pharmacy and Medical Sciences, 34 (1), 1–4. https://doi.org/10.2478/cipms-2021-0001
- Lapach, S. N. (2016). Rehressyonnii analyz. Protsessnii podkhod. Matematychni mashyny i systemy, 1, 125–133.
- Yamuna, M., Elakkiya, A. (2017). Mathematical Models in Drug Discovery, Development and Treatment of Various Diseases-A Case Study. Research Journal of Pharmacy and Technology, 10 (12), 4397-4401. https://doi.org/10.5958/0974-360x.2017.00810.1
- Hogg, R. V., McKean, J. W., Craig, A. T. (2019). Introduction to mathematical statistics. Boston: Pearson, 762.
- Salukvadze, M. E. (1979). Vector-valued optimization problems in control theory. Vol. 148 (Mathematics in Science and Engineering). Academic Press, 219.
- Pascoletti, A., Serafini, P. (1984). Scalarizing vector optimization problems. Journal of Optimization Theory and Applications, 42 (4), 499–524. https://doi.org/10.1007/bf00934564
- Kutova, O. V., Sahaidak-Nikitiuk, R. V. (2023). A methodical approach to the multi-criteria selection in the pharmaceutical technological research with quantitative factors. News of Pharmacy, 105 (1), 38–43. https://doi.org/10.24959/nphj.23.105
- Lapach, S. N., Radchenko, S. H., Babych, P. N. (1993). Planyrovanye, rehressyia y analyz modelei PRIAM (PRYAM). Kyiv, 24–27.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2024 Olga Kutova, Rita Sahaidak-Nikitiuk, Inna Kovalevska, Nataliya Demchenko

This work is licensed under a Creative Commons Attribution 4.0 International License.
Our journal abides by the Creative Commons CC BY copyright rights and permissions for open access journals.







