Construction of a mathematical model and numerical study of interaction between moisture-, heat-, and mass transfer processes of salt solutions in an unsaturated soil layer
DOI:
https://doi.org/10.15587/1729-4061.2022.265809Keywords:
moisture-, heat-, and mass transfer, aeration zone (incomplete saturation), method of finite differencesAbstract
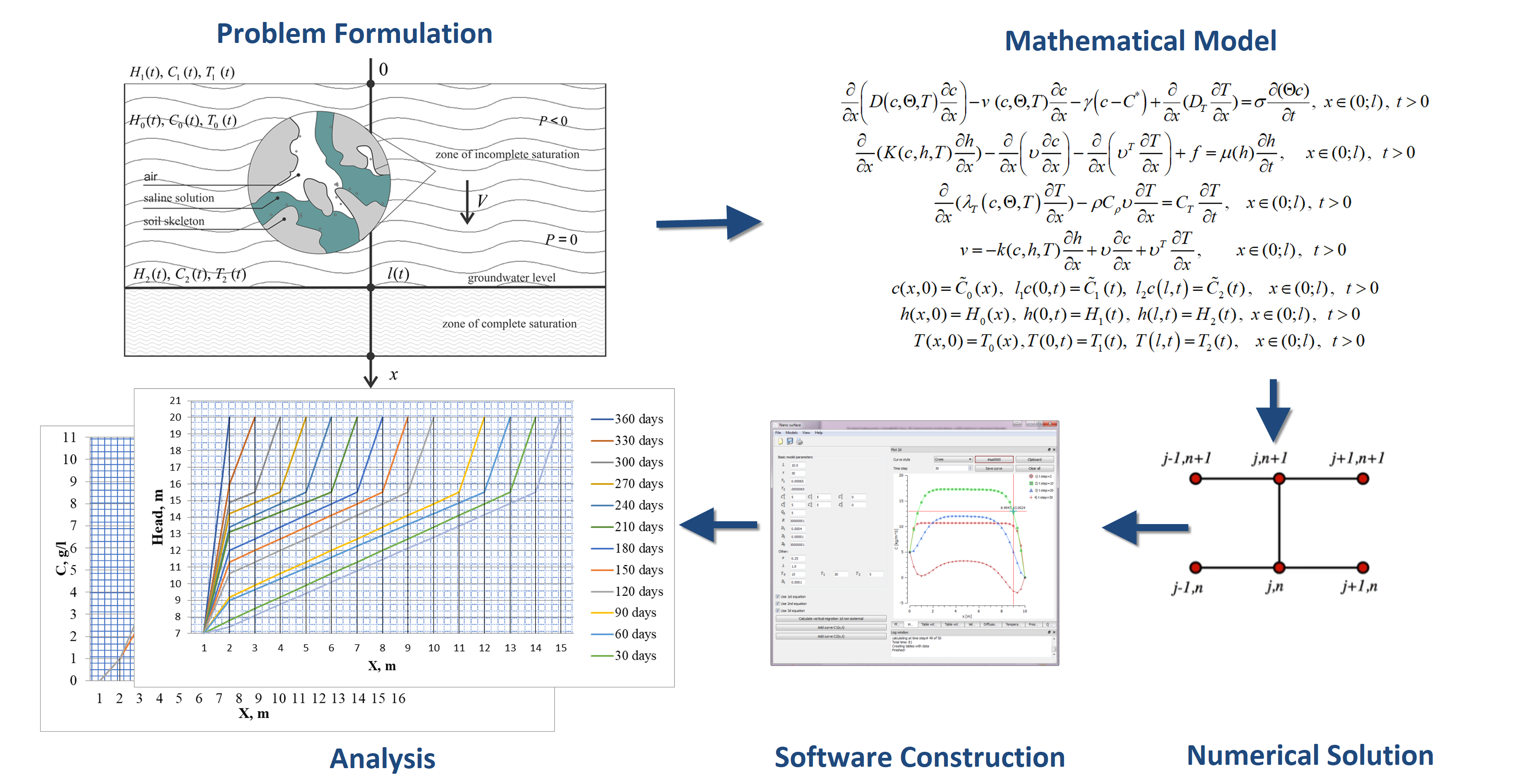
Methods of mathematical modeling and computer simulation were used to investigate the influence of interrelated processes of transfer of saline solutions in the unsaturated soil layer. To this end, a mathematical model has been built for modeling the corresponding processes of the moisture-, heat-, and mass transfer of saline solutions in an unsaturated soil layer. An effective computational algorithm was developed to solve the corresponding nonlinear boundary problem numerically by the method of finite differences; it was implemented in the Asp.net programming environment in the C++ language. Based on the numerical experiments carried out, the distribution of moisture, concentration, and temperature fields in the aeration zone (incomplete saturation) was obtained. To study the influence of mass transfer of salts on moisture transfer, a numerical solution was found to the problem of moisture transfer, the problem of moisture transfer taking into consideration mass transfer and moisture transfer, taking into consideration mass transfer in the presence of osmosis. Analysis of the results showed that the distribution of the concentration of saline solutions over time is slower and more predictable. It was established that the distribution of moisture heads increases with depth and time when saline solutions fall on the surface of the soil massif. With the influence of salt concentration, the distribution of moisture increases with depth and time throughout the entire area of moisture transfer by 1–3 %. The distribution of moisture heads taking into consideration the concentration of salts and osmosis is reduced by 3–5 % compared to the results of the problem without taking into consideration the phenomenon of osmosis. The distribution of the concentration of saline solutions during moisture transfer and osmosis acquires higher values compared to the results without taking osmosis into consideration. The established features can be successfully applied to clean the fertile soil layer and resume agricultural activities
References
- Çermikli, E., Şen, F., Altıok, E., Wolska, J., Cyganowski, P., Kabay, N., Bryjak, M., Arda, M., Yüksel, M. (2020). Performances of novel chelating ion exchange resins for boron and arsenic removal from saline geothermal water using adsorption-membrane filtration hybrid process. Desalination, 491, 114504. doi: https://doi.org/10.1016/j.desal.2020.114504
- Lekakis, E. H., Antonopoulos, V. Z. (2015). Modeling the effects of different irrigation water salinity on soil water movement, uptake and multicomponent solute transport. Journal of Hydrology, 530, 431–446. doi: https://doi.org/10.1016/j.jhydrol.2015.09.070
- Karwa, R. (2020). Heat and Mass Transfer. Publisher: Springer Nature. doi: https://doi.org/10.1007/978-981-15-3988-6
- Xu, F., Zhang, Y., Jin, G., Li, B., Kim, Y.-S., Xie, G., Fu, Z. (2018). Three phase heat and mass transfer model for unsaturated soil freezing process: Part 1 – model development. Open Physics, 16 (1), 75–83. doi: https://doi.org/10.1515/phys-2018-0014
- Zhang, Y., Xu, F., Li, B., Kim, Y.-S., Zhao, W., Xie, G., Fu, Z. (2018). Three phase heat and mass transfer model for unsaturated soil freezing process: Part 2 – model validation. Open Physics, 16 (1), 84–92. doi: https://doi.org/10.1515/phys-2018-0015
- Chen, Y., Lai, Y., Li, H., Pei, W. (2022). Finite element analysis of heat and mass transfer in unsaturated freezing soils: Formulation and verification. Computers and Geotechnics, 149, 104848. doi: https://doi.org/10.1016/j.compgeo.2022.104848
- Vlasiuk, A. P., Ilkiv, I. V. (2018). Matematychne ta kompiuterne modeliuvannia perenesennia solei pry filtratsii ta volohoperenesenni v nasycheno-nenasychenykh hruntakh v odnovymirnomu vypadku v neizotermichnykh umovakh. Suchasni problemy matematychnoho modeliuvannia, prohnozuvannia ta optymizatsii. Kamianets-Podilskyi, 22.
- Vlasyuk, A. P., Ilkiv, I. V. (2018). Mathematical modelling of salt transfer in horizontal non-saturated soil mass under nonisotermal conditions. Problems of decision making under uncertainties. Lancaran-Baku, 132.
- Vlasyuk, A. P., Zhukovskii, V. V. (2017). Mathematical Simulation of the Migration of Radionuclides in a Soil Medium Under Nonisothermal Conditions with Account for Catalytic Microparticles and Nonlinear Processes. Journal of Engineering Physics and Thermophysics, 90 (6), 1386–1398. doi: https://doi.org/10.1007/s10891-017-1697-4
- Vlasyuk, A. P., Tsvetkova, T. P. (2015). Mathematical Simulation of the Transport of Salt in the Case of Filtration and Moisture Transfer in Saturated–Unsaturated Soils in a Moistening Regime. Journal of Engineering Physics and Thermophysics, 88 (5), 1062–1073. doi: https://doi.org/10.1007/s10891-015-1285-4
- Vlasyuk, A., Tsvietkova, T., Ilkiv, I., Ogiychuk, V. (2021).Mathematical modelling of the infiltration impact on heart mass transfer in layered soils under conditions of heat transfer. Conference Paper 11th International Conference on Advanced Computer Information Technologies (ACIT2021), Deggendorf, 9–12. doi: https://doi.org/10.1109/acit52158.2021.9548542
- Zhangxin, C., Yuanle, M. (2006). Computational Methods for Multiphase Flows in Porous Media (Computational Science and Engineering). Paperback. Society for Industrial and Applied Mathematic, 531.
- Bear, J., Bachmat, Y. (2022). Introduction to modeling of Transport Phenomena in Porous Media. Dordrecht: Kluwer Academic Publishers, 580. doi: https://doi.org/10.1007/978-94-009-1926-6
- Kuzlo, M., Zhukovska, N., Zhukovskyy, V., Tarasyuk, N. (2020). Experimental Investigations of Filtration Coefficient of Clay Soils under Anthropogenic Factors Influence. 2020 IEEE 15th International Scientific and Technical Conference on Computer Sciences and Information Technologies, CSIT 2020 – Proceedings, 1, 411–414. doi: http://doi.org/10.1109/csit49958.2020.9321879
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2022 Ihor Ilkiv, Viktor Zhukovskyy, Nataliia Zhukovska, Andrii Safonyk

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.
A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.








