Constructing mathematical models of thermal conductivity in individual elements and units of electronic devices at local heating considering thermosensitivity

Authors

DOI:

https://doi.org/10.15587/1729-4061.2024.304804

Keywords:

temperature field, thermal conductivity of material, convective heat exchange, heat flow, thermal resistance of structures

Abstract

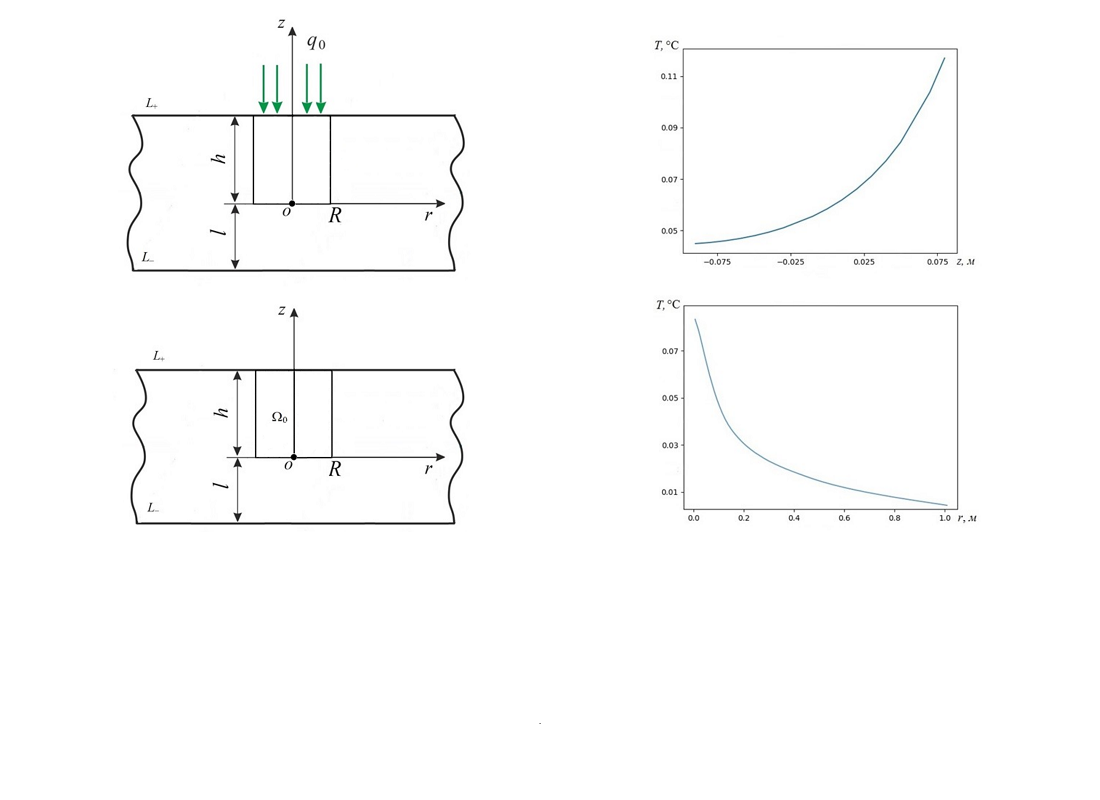
This paper considers a heat conduction process for an isotropic medium with local external and internal thermal heating. It was necessary to construct linear and non-linear mathematical models for determining the temperature field, and consequently, for the analysis of temperature regimes in these heat-active environments. To solve the linear boundary value problems and the resulting linearized boundary value problems with respect to the Kirchhoff transformation, the Henkel integral transformation method was used, as a result of which the analytical solutions to these problems were obtained. For a heat-sensitive environment, as an example, a linear dependence of the coefficient of thermal conductivity of the structural material of the structure on temperature, which is often used in many practical problems, was chosen. As a result, analytical relations for determining the temperature distribution in this environment were established. To determine the numerical values of the temperature and analyze the heat exchange processes in the given structure, caused by the external heat load, a geometric image of the temperature distribution was constructed depending on spatial coordinates. The resulting linear and non-linear mathematical models testify to their adequacy to the real physical process. They make it possible to analyze heat-active media regarding their thermal resistance. As a result, it becomes possible to increase it and protect it from overheating, which can cause the destruction of not only individual nodes and their elements but the entire structure as well

Author Biographies

Vasyl Havrysh, Lviv Polytechnic National University

Doctor of Technical Sciences, Professor

Department of Software

Elvira Dzhumelia, Lviv Polytechnic National University

PhD

Department of Software

Stepan Kachan, Lviv Polytechnic National University

PhD, Associate Professor

Department of Civil Safety

Pavlo Serdyuk, Lviv Polytechnic National University

PhD, Associate Professor

Department of Software

Viktoria Maikher, Lviv Polytechnic National University

PhD

Department of Software

References

  1. Haopeng, S., Kunkun, X., Cunfa, G. (2021). Temperature, thermal flux and thermal stress distribution around an elliptic cavity with temperature-dependent material properties. International Journal of Solids and Structures, 216, 136–144. https://doi.org/10.1016/j.ijsolstr.2021.01.010
  2. Zhang, Z., Zhou, D., Fang, H., Zhang, J., Li, X. (2021). Analysis of layered rectangular plates under thermo-mechanical loads considering temperature-dependent material properties. Applied Mathematical Modelling, 92, 244–260. https://doi.org/10.1016/j.apm.2020.10.036
  3. Gong, J., Xuan, L., Ying, B., Wang, H. (2019). Thermoelastic analysis of functionally graded porous materials with temperature-dependent properties by a staggered finite volume method. Composite Structures, 224, 111071. https://doi.org/10.1016/j.compstruct.2019.111071
  4. Demirbas, M. D. (2017). Thermal stress analysis of functionally graded plates with temperature-dependent material properties using theory of elasticity. Composites Part B: Engineering, 131, 100–124. https://doi.org/10.1016/j.compositesb.2017.08.005
  5. Ghannad, M., Yaghoobi, M. P. (2015). A thermoelasticity solution for thick cylinders subjected to thermo-mechanical loads under various boundary conditions. International Journal of Advanced Design and Manufacturing Technology, 8 (4). Available at: https://sanad.iau.ir/journal/admt/Article/534941?jid=534941
  6. Parhizkar Yaghoobi, M., Ghannad, M. (2020). An analytical solution for heat conduction of FGM cylinders with varying thickness subjected to non-uniform heat flux using a first-order temperature theory and perturbation technique. International Communications in Heat and Mass Transfer, 116, 104684. https://doi.org/10.1016/j.icheatmasstransfer.2020.104684
  7. Eker, M., Yarımpabuç, D., Çelebi, K. (2020). Thermal stress analysis of functionally graded solid and hollow thick-walled structures with heat generation. Engineering Computations, 38 (1), 371–391. https://doi.org/10.1108/ec-02-2020-0120
  8. Bayat, A., Moosavi, H., Bayat, Y. (2015). Thermo-mechanical analysis of functionally graded thick spheres with linearly time-dependent temperature. Scientia Iranica, 22 (5), 1801–1812. Available at: https://scientiairanica.sharif.edu/article_3743.html
  9. Evstatieva, N., Evstatiev, B. (2023). Modelling the Temperature Field of Electronic Devices with the Use of Infrared Thermography. 2023 13th International Symposium on Advanced Topics in Electrical Engineering (ATEE). https://doi.org/10.1109/atee58038.2023.10108375
  10. Liu, H., Yu, J., Wang, R. (2023). Dynamic compact thermal models for skin temperature prediction of portable electronic devices based on convolution and fitting methods. International Journal of Heat and Mass Transfer, 210, 124170. https://doi.org/10.1016/j.ijheatmasstransfer.2023.124170
  11. Bianco, V., De Rosa, M., Vafai, K. (2022). Phase-change materials for thermal management of electronic devices. Applied Thermal Engineering, 214, 118839. https://doi.org/10.1016/j.applthermaleng.2022.118839
  12. Mathew, J., Krishnan, S. (2021). A Review On Transient Thermal Management of Electronic Devices. Journal of Electronic Packaging. https://doi.org/10.1115/1.4050002
  13. Zhou, K., Ding, H., Steenbergen, M., Wang, W., Guo, J., Liu, Q. (2021). Temperature field and material response as a function of rail grinding parameters. International Journal of Heat and Mass Transfer, 175, 121366. https://doi.org/10.1016/j.ijheatmasstransfer.2021.121366
  14. Liu, X., Peng, W., Gong, Z., Zhou, W., Yao, W. (2022). Temperature field inversion of heat-source systems via physics-informed neural networks. Engineering Applications of Artificial Intelligence, 113, 104902. https://doi.org/10.1016/j.engappai.2022.104902
  15. Kong, Q., Jiang, G., Liu, Y., Yu, M. (2020). Numerical and experimental study on temperature field reconstruction based on acoustic tomography. Applied Thermal Engineering, 170, 114720. https://doi.org/10.1016/j.applthermaleng.2019.114720
  16. Havrysh, V., Kochan, V. (2023). Mathematical Models to Determine Temperature Fields in Heterogeneous Elements of Digital Devices with Thermal Sensitivity Taken into Account. 2023 IEEE 12th International Conference on Intelligent Data Acquisition and Advanced Computing Systems: Technology and Applications (IDAACS). https://doi.org/10.1109/idaacs58523.2023.10348875
  17. Havrysh, V. I., Kolyasa, L. I., Ukhanska, O. M., Loik, V. B. (2019). Determination of temperature field in thermally sensitive layered medium with inclusions. Naukovyi Visnyk Natsionalnoho Hirnychoho Universytetu, 1, 76–82. https://doi.org/10.29202/nvngu/2019-1/5
  18. Havrysh, V., Ovchar, I., Baranetskyj, J., Pelekh, J., Serduik, P. (2017). Development and analysis of mathematical models for the process of thermal conductivity for piecewise uniform elements of electronic systems. Eastern-European Journal of Enterprise Technologies, 1 (5 (85)), 23–33. https://doi.org/10.15587/1729-4061.2017.92551
  19. Havrysh, V. I., Kosach, A. I. (2012). Boundary-value problem of heat conduction for a piecewise homogeneous layer with foreign inclusion. Materials Science, 47 (6), 773–782. https://doi.org/10.1007/s11003-012-9455-4
  20. Gavrysh, V., Tushnytskyy, R., Pelekh, Y., Pukach, P., Baranetskyi, Y. (2017). Mathematical model of thermal conductivity for piecewise homogeneous elements of electronic systems. 2017 14th International Conference The Experience of Designing and Application of CAD Systems in Microelectronics (CADSM). https://doi.org/10.1109/cadsm.2017.7916146
Constructing mathematical models of thermal conductivity in individual elements and units of electronic devices at local heating considering thermosensitivity

Downloads

Published

2024-06-28

How to Cite

Havrysh, V., Dzhumelia, E., Kachan, S., Serdyuk, P., & Maikher, V. (2024). Constructing mathematical models of thermal conductivity in individual elements and units of electronic devices at local heating considering thermosensitivity. Eastern-European Journal of Enterprise Technologies, 3(5 (129), 25–35. https://doi.org/10.15587/1729-4061.2024.304804

Issue

Vol. 3 No. 5 (129) (2024): Applied physics

Section

Applied physics

License

Copyright (c) 2024 Vasyl Havrysh, Elvira Dzhumelia, Stepan Kachan, Pavlo Serdyuk, Viktoria Maikher

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.

A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.