Optimization of cross-sectional dimensions of castellated beams with hexagonal openings
DOI:
https://doi.org/10.15587/1729-4061.2024.304803Keywords:
castellated beam, hexagonal openings, optimal design, mixed variables, exhaustive searchAbstract
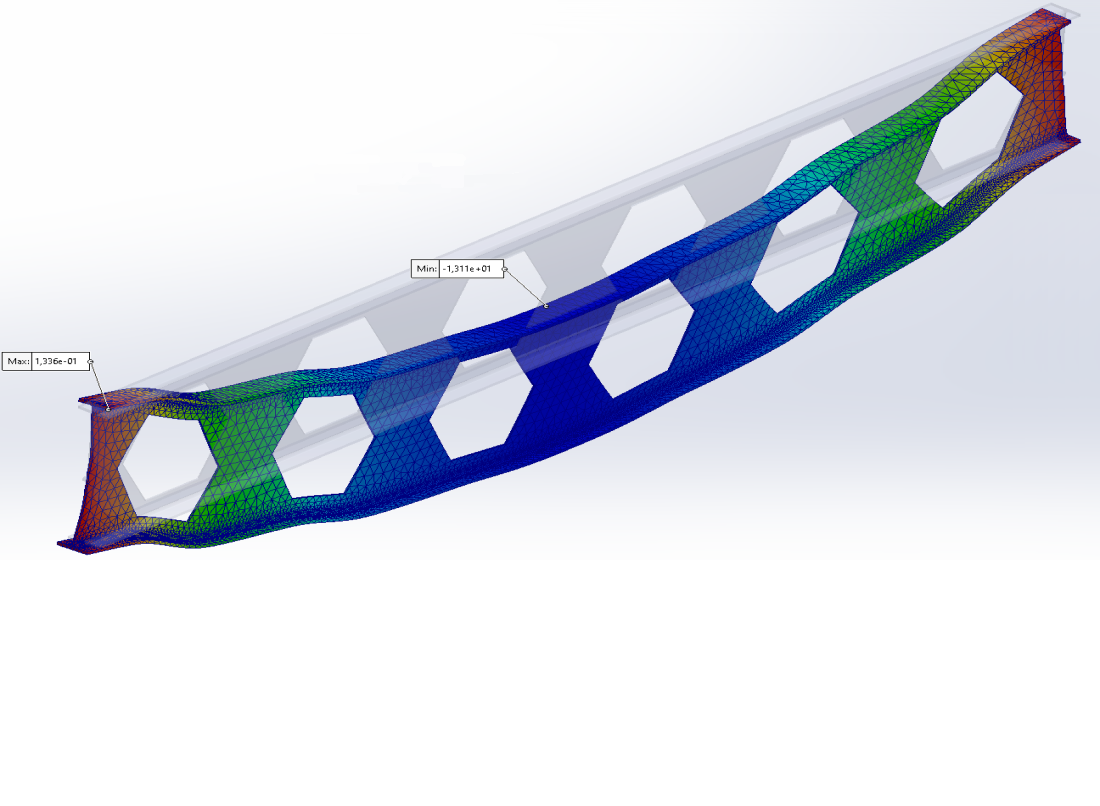
The object of this study is a castellated beam, in which the web openings have the shape of a regular hexagon. The beam is examined to find optimal cross-sectional dimensions. The optimization task is stated as the task of finding the optimal profile numbers for top and bottom Tees of the beam and the optimal width of the web opening while ensuring the required load-carrying capacity of the beam. Minimization of the volume of the beam material was considered as an optimality criterion. The stated optimization problem was solved using the exhaustive search method. For an assortment of normal I-beams with parallel flanges, castellated beams were obtained with optimal cross-sectional dimensions depending on the steel grade, the beam span, and the magnitude of transverse uniformly distributed load. The optimization calculations proved that it was possible to increase the elastic section modulus of the beam to 35.48...50 % through the use of a castellated web. Castellated beams with optimal cross-sectional dimensions at the same load-carrying capacity are characterized by lower steel consumption (up to 23.19 %) compared to I-beams with a solid web. Analysis of the results has made it possible to devise recommendations for the optimal distribution of material in the cross-sections of such beams. The results are valid only for the assortment of normal I-beam profiles and only for the case of uniformly distributed load acting on the beam when the compressed beam flange is laterally restrained from the bending plane and the beam web has openings in the form of regular hexagons. It is under such conditions that the reported results can be implemented in practice both at the stage of selecting cross-sections of the studied class of structures, and at the development of effective assortments of castellated beams
References
- Pavlović, S. (2021). Techno-economic analysis of castellated and solid "I"- profiled steel beams in terms of load capacity and serviceability. STEPGRAD, 1 (13). https://doi.org/10.7251/stp1813739p
- Gezentsvey, Y., Olevskyi, V., Volchok, D., Olevskyi, O. (2021). Calculation of the improved steel beams of buildings and structures of the mining and metallurgical complex. Strength of Materials and Theory of Structures, 106, 54–67. https://doi.org/10.32347/2410-2547.2021.106.54-67
- Mahdi, A. S., Alshimmeri, A. J. H. (2023). Analytical study of castellated steel beams with and without strengthening web. AIP Conference Proceedings. https://doi.org/10.1063/5.0171338
- Yurchenko, V., Peleshko, I. (2021). Methodology for solving parametric optimization problems of steel structures. Magazine of Civil Engineering, 7 (107). https://doi.org/10.34910/MCE.107.5
- Poul, S., Mote, P. S. (2022). Optimization for various parameters of castellated beam containing sinusoidal openings. Journal of emerging technologies and innovative research, 9 (2), a253–a258. Available at: https://www.ijert.org/research/optimization-of-various-parameters-of-castellated-beam-containing-sinusoidal-openings-IJERTV10IS060008.pdf
- Kurlapkar, R., Patil, A. (2021). Optimization of various parameters of castellated beam containing sinusoidal openings. International journal of engineering research & technology, 10 (06), 120–123. Available at: https://www.ijert.org/research/optimization-of-various-parameters-of-castellated-beam-containing-sinusoidal-openings-IJERTV10IS060008.pdf
- Budi, L., Sukamta, Partono, W. (2017). Optimization Analysis of Size and Distance of Hexagonal Hole in Castellated Steel Beams. Procedia Engineering, 171, 1092–1099. https://doi.org/10.1016/j.proeng.2017.01.465
- Kaveh, A., Almasi, P., Khodagholi, A. (2022). Optimum Design of Castellated Beams Using Four Recently Developed Meta-heuristic Algorithms. Iranian Journal of Science and Technology, Transactions of Civil Engineering, 47 (2), 713–725. https://doi.org/10.1007/s40996-022-00884-z
- Yang, X. (2010). Engineering Optimization. John Wiley & Sons, Inc. https://doi.org/10.1002/9780470640425
- Kaveh, A., Kaveh, A., Shokohi, F. (2016). A hybrid optimization algorithm for the optimal design of laterally-supported castellated beams. Scientia Iranica, 23 (2), 508–519. https://doi.org/10.24200/sci.2016.2135
- Kaveh, A., Shokohi, F. (2015). Optimum design of laterally-supported castellated beams using CBO algorithm. Steel and Composite Structures, 18 (2), 305–324. https://doi.org/10.12989/scs.2015.18.2.305
- Erdal, F., Doğan, E., Saka, M. P. (2011). Optimum design of cellular beams using harmony search and particle swarm optimizers. Journal of Constructional Steel Research, 67 (2), 237–247. https://doi.org/10.1016/j.jcsr.2010.07.014
- Sorkhabi, R. V., Naseri, A., Naseri, M. (2014). Optimization of the Castellated Beams by Particle Swarm Algorithms Method. APCBEE Procedia, 9, 381–387. https://doi.org/10.1016/j.apcbee.2014.01.067
- Kaveh, A. (2014). Cost optimization of castellated beams using charged system search algorithm. Iranian Journal of Science and Technology - Transactions of Civil Engineering, 38 (C1+), 235–249. Available at: https://www.researchgate.net/publication/266933455_Cost_optimization_of_castellated_beams_using_charged_system_search_algorithm#fullTextFileContent
- Kaveh, A., Shokohi, F. (2016). Application of grey wolf optimizer in design of castellated beams. Asian Journal of Civil Engineering (BHRC), 17 (5), 683–700. Available at: https://www.researchgate.net/publication/287865960_Application_of_Grey_Wolf_Optimizer_in_design_of_castellated_beams#fullTextFileContent
- Permyakov, V. O., Yurchenko, V. V., Peleshko, I. D. (2006). An optimum structural computer-aided design using hybrid genetic algorithm. Proceeding of the International Conference “Progress in Steel, Composite and Aluminium Structures”, 819–826. Available at: https://www.researchgate.net/publication/318040068_An_optimum_structural_computer-aided_design_using_hybrid_genetic_algorithm#fullTextFileContent
- Tanady, K., Suryoatmono, B. (2024). Numerical Study of Behavior of Castellated Beam under Cyclic Loading. Civil Engineering and Architecture, 12 (1), 185–202. https://doi.org/10.13189/cea.2024.120116
- Soltani, M. R., Bouchaïr, A., Mimoune, M. (2012). Nonlinear FE analysis of the ultimate behavior of steel castellated beams. Journal of Constructional Steel Research, 70, 101–114. https://doi.org/10.1016/j.jcsr.2011.10.016
- Morkhade, S. G., Gupta, L. M. (2019). Behavior of Castellated Steel Beams: State of the Art Review. Electronic Journal of Structural Engineering, 19, 39–48. https://doi.org/10.56748/ejse.19234
- Elaiwi, S. S., Kim, B., Li, L. (2019). Linear and Nonlinear Buckling Analysis of Castellated Beams. International Journal of Structural and Civil Engineering Research, 8 (2), 83–93. https://doi.org/10.18178/ijscer.8.2.83-93
- Peleshko, I. D., Yurchenko, V. V. (2021). Parametric Optimization of Metal Rod Structures Using the Modified Gradient Projection Method. International Applied Mechanics, 57 (4), 440–454. https://doi.org/10.1007/s10778-021-01096-0
- Yurchenko, V. V., Peleshko, I. D., Biliaiev, N. (2021). Application of improved gradient projection method to parametric optimization of steel lattice portal frame. IOP Conference Series: Materials Science and Engineering, 1164 (1), 012090. https://doi.org/10.1088/1757-899x/1164/1/012090
- Jiang, T.-Y., Xu, M.-X., Geng, S., Wang, L. (2024). Calculation of deformation behavior and deflection of regular hexagonal castellated beams considering web weld damage. Engineering Mechanics, 41 (4), 199–209. https://doi.org/10.6052/j.issn.1000-4750.2022.04.0382
- Perelmuter, A., Kriksunov, E., Gavrilenko, I., Yurchenko, V. (2010). Designing bolted end-plate connections in compliance with Eurocode and Ukrainian codes: consistency and contradictions. Selected papers of the 10th International Conference “Modern Building Materials, Structures and Techniques”. Vol. II. Vilnius: Technika, 733–743. Available at: https://www.researchgate.net/publication/266038627_Designing_bolted_end-plate_connections_in_compliance_with_eurocode_and_ukrainian_codes_Consistency_and_contradictions
- Yurchenko, V., Peleshko, I. (2022). Optimization of cross-section dimensions of structural members made of cold-formed profiles using compromise search. Eastern-European Journal of Enterprise Technologies, 5 (7 (119)), 84–95. https://doi.org/10.15587/1729-4061.2022.261037
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2024 Vitalina Yurchenko, Ivan Peleshko, Pavlo Rusyn

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.
A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.








