Розробка моделі механізму подрібнення ударом в барабанному млині на основі візуалізації даних

Автор(и)

  • Юрій Васильович Науменко Національний університет водного господарства та природокористування, Україна https://orcid.org/0000-0003-3658-3087
  • Катерина Юріївна Дейнека Національний університет водного господарства та природокористування, Україна https://orcid.org/0000-0001-7376-6734

DOI:

https://doi.org/10.15587/1729-4061.2023.283073

Ключові слова:

барабанний млин, внутрішньокамерне завантаження, ударна дія, зона польоту, критерії подібності, продуктивність подрібнення

Анотація

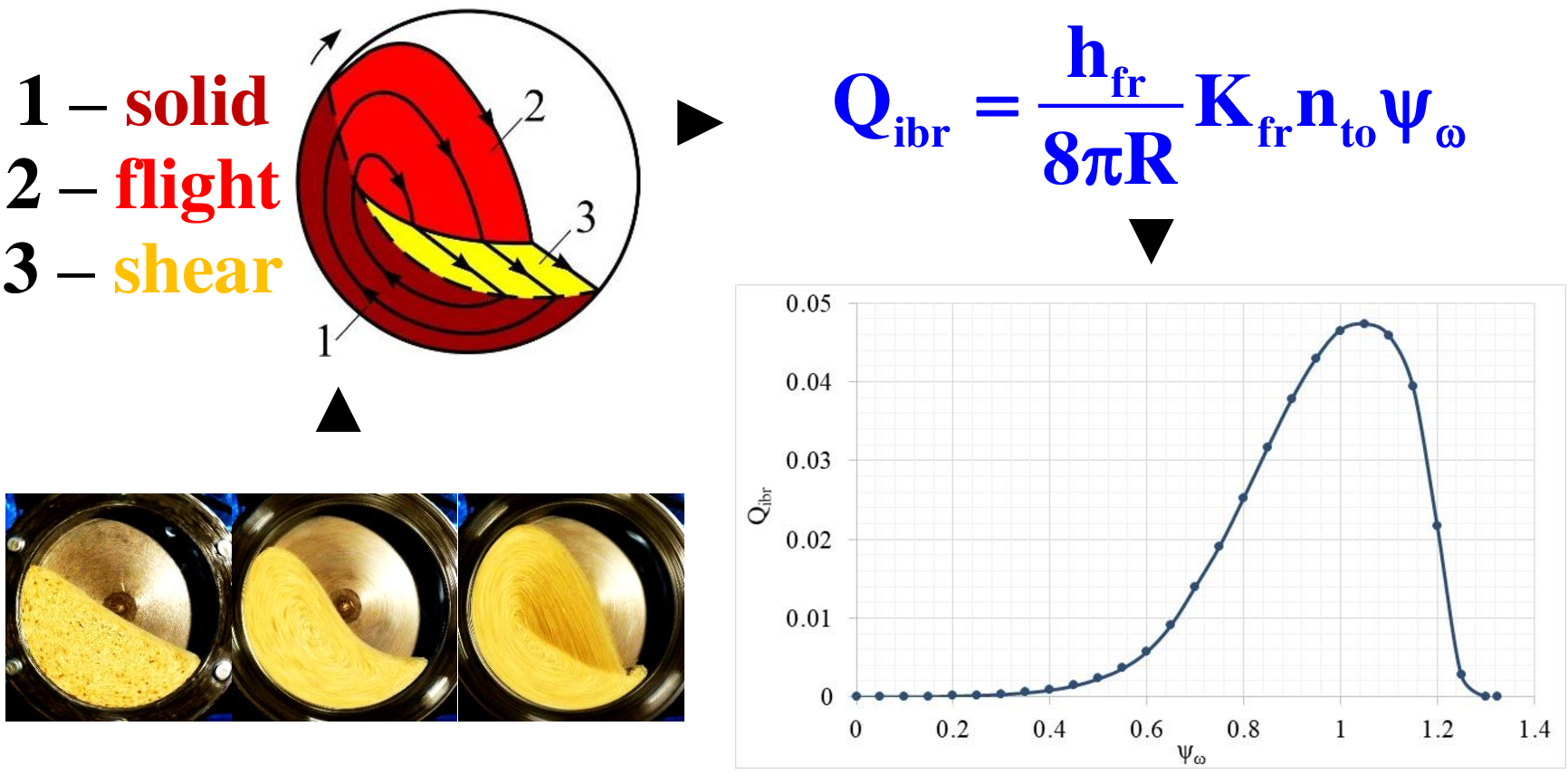
Побудовано математичну модель на основі візуалізації даних для механізму подрібнення ударом в барабанному млині, що реалізується переважно при грубому помелі.

Визначення параметрів імпульсної взаємодії є проблематичним через труднощі моделювання та складність апаратурного аналізу поведінки внутрішньомлинного завантаження.

Концептуально передбачалось виявлення відносних динамічних параметрів ударної дії як складових моделі, що є критеріями подібності руху завантаження та процесу помелу. Як аналог продуктивності подрібнення прийнято потужність ударних сил. Вихідною характеристикою удару вважалась усереднена вертикальна складова швидкості руху завантаження у зоні польоту на межі контакту зі зсувним шаром. Формалізація моделі виявила вплив на продуктивність масової частки зони польоту та оборотності завантаження.

Застосовано метод чисельного моделювання на основі експериментальної візуалізації поведінки зернистого завантаження в поперечному перерізі обертової камери.

Експериментальним моделюванням оцінено вплив швидкості обертання на продуктивність при ступені заповнення камери 0.45 та відносному розмірі частинок молольного завантаження 0.0104. Виявлено максимальне значення продуктивності при відносній швидкості обертання ψω=1–1.05. Встановлено раціональну умову реалізації подрібнення ударом при ψω=0.75–0.9.

Апробація засвідчила ефективність застосування візуалізації для оцінювання динамічних аналогів взаємодії завантаження. Верифікація результатів моделювання реалізована порівнянням із даними технічного стандарту. Використання критеріїв подібності уніфікує підходи до моделювання різних механізмів руйнування.

Розроблена модель дозволяє прогнозувати раціональні параметри процесів подрібнення ударом, роздавлюванням та стиранням

Біографії авторів

Юрій Васильович Науменко, Національний університет водного господарства та природокористування

Доктор технічних наук, доцент

Кафедра будівельних, дорожніх, меліоративних, сільськогосподарських машин і обладнання

Катерина Юріївна Дейнека, Національний університет водного господарства та природокористування

Кандидат технічних наук, викладач вищої категорії

Рівненський технічний фаховий коледж

Посилання

  1. Fuerstenau, D. W., Abouzeid, A.-Z. M. (2002). The energy efficiency of ball milling in comminution. International Journal of Mineral Processing, 67 (1-4), 161–185. doi: https://doi.org/10.1016/s0301-7516(02)00039-x
  2. Tromans, D. (2008). Mineral comminution: Energy efficiency considerations. Minerals Engineering, 21 (8), 613–620. doi: https://doi.org/10.1016/j.mineng.2007.12.003
  3. Napier-Munn, T. (2015). Is progress in energy-efficient comminution doomed? Minerals Engineering, 73, 1–6. doi: https://doi.org/10.1016/j.mineng.2014.06.009
  4. Bouchard, J., LeBlanc, G., Levesque, M., Radziszewski, P., Georges-Filteau, D. (2019). Breaking down energy consumption in industrial grinding mills. CIM Journal, 10 (4), 157–164. doi: https://doi.org/10.15834/cimj.2019.18
  5. Chimwani, N. (2021). A Review of the Milestones Reached by the Attainable Region Optimisation Technique in Particle Size Reduction. Minerals, 11 (11), 1280. doi: https://doi.org/10.3390/min11111280
  6. Cleary, P. W. (2001). Charge behaviour and power consumption in ball mills: sensitivity to mill operating conditions, liner geometry and charge composition. International Journal of Mineral Processing, 63 (2), 79–114. doi: https://doi.org/10.1016/s0301-7516(01)00037-0
  7. Morrison, R. D., Cleary, P. W. (2008). Towards a virtual comminution machine. Minerals Engineering, 21 (11), 770–781. doi: https://doi.org/10.1016/j.mineng.2008.06.005
  8. Bilgili, E., Scarlett, B. (2005). Population balance modeling of non-linear effects in milling processes. Powder Technology, 153 (1), 59–71. doi: https://doi.org/10.1016/j.powtec.2005.02.005
  9. Wills, B. A., Finch, J. (2015). Wills' mineral processing technology: An introduction to the practical aspects of ore treatment and mineral recovery. Butterworth-Heinemann. doi: https://doi.org/10.1016/c2010-0-65478-2
  10. Gupta, V. K. (2020). Energy absorption and specific breakage rate of particles under different operating conditions in dry ball milling. Powder Technology, 361, 827–835. doi: https://doi.org/10.1016/j.powtec.2019.11.033
  11. Datta, A., Mishra, B. K. (1999). Power draw estimation of ball mills using neural networks. Mining, Metallurgy & Exploration, 16 (1), 57–60. doi: https://doi.org/10.1007/bf03402857
  12. Rezaeizadeh, M., Fooladi, M., Powell, M. S., Mansouri, S. H. (2010). Experimental observations of lifter parameters and mill operation on power draw and liner impact loading. Minerals Engineering, 23 (15), 1182–1191. doi: https://doi.org/10.1016/j.mineng.2010.07.017
  13. Soleymani, M. M., Fooladi, M., Rezaeizadeh, M. (2016). Experimental investigation of the power draw of tumbling mills in wet grinding. Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 230 (15), 2709–2719. doi: https://doi.org/10.1177/0954406215598801
  14. Góralczyk, M., Krot, P., Zimroz, R., Ogonowski, S. (2020). Increasing Energy Efficiency and Productivity of the Comminution Process in Tumbling Mills by Indirect Measurements of Internal Dynamics—An Overview. Energies, 13 (24), 6735. doi: https://doi.org/10.3390/en13246735
  15. Golpayegani, M. H., Rezai, B. (2022). Modelling the power draw of tumbling mills: A comprehensive review. Physicochemical Problems of Mineral Processing. doi: https://doi.org/10.37190/ppmp/151600
  16. Govender, I Powell, M. S. (2006). An empirical power model derived from 3D particle tracking experiments. Minerals Engineering, 19 (10), 1005–1012. doi: https://doi.org/10.1016/j.mineng.2006.03.017
  17. Bbosa, L. S., Govender, I., Mainza, A. N., Powell, M. S. (2011). Power draw estimations in experimental tumbling mills using PEPT. Minerals Engineering, 24 (3-4), 319–324. doi: https://doi.org/10.1016/j.mineng.2010.10.005
  18. Bbosa, L. S., Govender, I., Mainza, A. (2016). Development of a novel methodology to determine mill power draw. International Journal of Mineral Processing, 149, 94–103. doi: https://doi.org/10.1016/j.minpro.2016.02.009
  19. Tohry, A., Chehreh Chelgani, S., Matin, S. S., Noormohammadi, M. (2020). Power-draw prediction by random forest based on operating parameters for an industrial ball mill. Advanced Powder Technology, 31 (3), 967–972. doi: https://doi.org/10.1016/j.apt.2019.12.012
  20. Tavares, L. M. (2017). A Review of Advanced Ball Mill Modelling. KONA Powder and Particle Journal, 34, 106–124. doi: https://doi.org/10.14356/kona.2017015
  21. Kelly, E. G., Spottiswood, D. J. (1982). Introduction to mineral processing. Wiley- Interscience.
  22. King, R. P. (2001). Modeling and simulation of mineral processing systems. Elsevier. doi: https://doi.org/10.1016/c2009-0-26303-3
  23. Chieregati, A. C., Delboni Júnior, H. (2001). Novo método de caracterização tecnológica para cominuição de minérios. São Paulo: EPUSP.
  24. Gupta, A., Yan, D. (2016). Mineral processing design and operations: An introduction. Elsevier. doi: https://doi.org/10.1016/c2014-0-01236-1
  25. Yin, Z., Peng, Y., Zhu, Z., Yu, Z., Li, T. (2017). Impact Load Behavior between Different Charge and Lifter in a Laboratory-Scale Mill. Materials, 10 (8), 882. doi: https://doi.org/10.3390/ma10080882
  26. Boateng, A. A., Barr, P. V. (1996). Modelling of particle mixing and segregation in the transverse plane of a rotary kiln. Chemical Engineering Science, 51 (17), 4167–4181. doi: https://doi.org/10.1016/0009-2509(96)00250-3
  27. Ding, Y. L., Forster, R., Seville, J. P. K., Parker, D. J. (2002). Granular motion in rotating drums: bed turnover time and slumping–rolling transition. Powder Technology, 124 (1-2), 18–27. doi: https://doi.org/10.1016/s0032-5910(01)00486-7
  28. Mellmann, J. (2001). The transverse motion of solids in rotating cylinders—forms of motion and transition behavior. Powder Technology, 118 (3), 251–270. doi: https://doi.org/10.1016/s0032-5910(00)00402-2
  29. Rajchenbach, J. (1990). Flow in powders: From discrete avalanches to continuous regime. Physical Review Letters, 65 (18), 2221–2224. doi: https://doi.org/10.1103/physrevlett.65.2221
  30. Zik, O., Levine, D., Lipson, S. G., Shtrikman, S., Stavans, J. (1994). Rotationally Induced Segregation of Granular Materials. Physical Review Letters, 73 (5), 644–647. doi: https://doi.org/10.1103/physrevlett.73.644
  31. Orpe, A. V., Khakhar, D. V. (2001). Scaling relations for granular flow in quasi-two-dimensional rotating cylinders. Physical Review E, 64 (3). doi: https://doi.org/10.1103/physreve.64.031302
  32. Taberlet, N., Richard, P., Hinch, E. J. (2006). S shape of a granular pile in a rotating drum. Physical Review E, 73 (5), 050301. doi: https://doi.org/10.1103/physreve.73.050301
  33. Govender, I., Richter, M. C., Mainza, A. N., De Klerk, D. N. (2016). A positron emission particle tracking investigation of the scaling law governing free surface flows in tumbling mills. AIChE Journal, 63 (3), 903–913. doi: https://doi.org/10.1002/aic.15453
  34. Yin, Z., Peng, Y., Li, T., Wu, G. (2018). DEM Investigation of Mill Speed and Lifter Face Angle on Charge Behavior in Ball Mills. IOP Conference Series: Materials Science and Engineering, 394, 032084. doi: https://doi.org/10.1088/1757-899x/394/3/032084
  35. Maleki-Moghaddam, M., Yahyaei, M., Banisi, S. (2013). A method to predict shape and trajectory of charge in industrial mills. Minerals Engineering, 46-47, 157–166. doi: https://doi.org/10.1016/j.mineng.2013.04.013
  36. Powell, M. S McBride, A. T. (2004). A three-dimensional analysis of media motion and grinding regions in mills. Minerals Engineering, 17 (11-12), 1099–1109. doi: https://doi.org/10.1016/j.mineng.2004.06.022
  37. Morrison, A. J., Govender, I., Mainza, A. N., Parker, D. J. (2016). The shape and behaviour of a granular bed in a rotating drum using Eulerian flow fields obtained from PEPT. Chemical Engineering Science, 152, 186–198. doi: https://doi.org/10.1016/j.ces.2016.06.022
  38. de Klerk, D. N., Govender, I., Mainza, A. N. (2019). Geometric features of tumbling mill flows: A positron emission particle tracking investigation. Chemical Engineering Science, 206, 41–49. doi: https://doi.org/10.1016/j.ces.2019.05.020
  39. Naumenko, Y. (2017). Modeling a flow pattern of the granular fill in the cross section of a rotating chamber. Eastern-European Journal of Enterprise Technologies, 5 (1 (89)), 59–69. doi: https://doi.org/10.15587/1729-4061.2017.110444
  40. Naumenko, Y. (2017). Modeling of fracture surface of the quasi solid-body zone of motion of the granular fill in a rotating chamber. Eastern-European Journal of Enterprise Technologies, 2 (1 (86)), 50–57. doi: https://doi.org/10.15587/1729-4061.2017.96447
  41. Naumenko, Y., Sivko, V. (2017). The rotating chamber granular fill shear layer flow simulation. Eastern-European Journal of Enterprise Technologies, 4 (7 (88)), 57–64. doi: https://doi.org/10.15587/1729-4061.2017.107242
  42. Deineka, K., Naumenko, Y. (2019). Revealing the effect of decreased energy intensity of grinding in a tumbling mill during self-excitation of auto-oscillations of the intrachamber fill. Eastern-European Journal of Enterprise Technologies, 1 (1), 6–15. doi: https://doi.org/10.15587/1729-4061.2019.155461
  43. Deineka, K., Naumenko, Y. (2020). Establishing the effect of decreased power intensity of self-oscillatory grinding in a tumbling mill when the crushed material content in the intra-chamber fill is reduced. Eastern-European Journal of Enterprise Technologies, 4 (1 (106)), 39–48. doi: https://doi.org/10.15587/1729-4061.2020.209050
  44. Deineka, K., Naumenko, Y. (2019). Establishing the effect of a decrease in power intensity of self-oscillating grinding in a tumbling mill with a reduction in an intrachamber fill. Eastern-European Journal of Enterprise Technologies, 6 (7 (102)), 43–52. doi: https://doi.org/10.15587/1729-4061.2019.183291
  45. Deineka, K., Naumenko, Y. (2021). Establishing the effect of a simultaneous reduction in the filling load inside a chamber and in the content of the crushed material on the energy intensity of self-oscillatory grinding in a tumbling mill. Eastern-European Journal of Enterprise Technologies, 1 (1 (109)), 77–87. doi: https://doi.org/10.15587/1729-4061.2021.224948
  46. Deineka, K., Naumenko, Y. (2022). Revealing the mechanism of stability loss of a two-fraction granular flow in a rotating drum. Eastern-European Journal of Enterprise Technologies, 4 (1 (118)), 34–46. doi: https://doi.org/10.15587/1729-4061.2022.263097
  47. Deineka, K. Yu., Naumenko, Yu. V. (2018). The tumbling mill rotation stability. Scientific Bulletin of National Mining University, 1, 60–68. doi: https://doi.org/10.29202/nvngu/2018-1/10
Розробка моделі механізму подрібнення ударом в барабанному млині на основі візуалізації даних

##submission.downloads##

Опубліковано

2023-06-30

Як цитувати

Науменко, Ю. В., & Дейнека, К. Ю. (2023). Розробка моделі механізму подрібнення ударом в барабанному млині на основі візуалізації даних. Eastern-European Journal of Enterprise Technologies, 3(7 (123), 65–73. https://doi.org/10.15587/1729-4061.2023.283073

Номер

Том 3 № 7 (123) (2023): Прикладна механіка

Розділ

Прикладна механіка

Ліцензія

Авторське право (c) 2023 Yuriy Naumenko, Kateryna Deineka

Creative Commons License

Ця робота ліцензується відповідно до Creative Commons Attribution 4.0 International License.

Закріплення та умови передачі авторських прав (ідентифікація авторства) здійснюється у Ліцензійному договорі. Зокрема, автори залишають за собою право на авторство свого рукопису та передають журналу право першої публікації цієї роботи на умовах ліцензії Creative Commons CC BY. При цьому вони мають право укладати самостійно додаткові угоди, що стосуються неексклюзивного поширення роботи у тому вигляді, в якому вона була опублікована цим журналом, але за умови збереження посилання на першу публікацію статті в цьому журналі.

Ліцензійний договір – це документ, в якому автор гарантує, що володіє усіма авторськими правами на твір (рукопис, статтю, тощо).
Автори, підписуючи Ліцензійний договір з ПП «ТЕХНОЛОГІЧНИЙ ЦЕНТР», мають усі права на подальше використання свого твору за умови посилання на наше видання, в якому твір опублікований. Відповідно до умов Ліцензійного договору, Видавець ПП «ТЕХНОЛОГІЧНИЙ ЦЕНТР» не забирає ваші авторські права та отримує від авторів дозвіл на використання та розповсюдження публікації через світові наукові ресурси (власні електронні ресурси, наукометричні бази даних, репозитарії, бібліотеки тощо).
За відсутності підписаного Ліцензійного договору або за відсутністю вказаних в цьому договорі ідентифікаторів, що дають змогу ідентифікувати особу автора, редакція не має права працювати з рукописом.
Важливо пам’ятати, що існує і інший тип угоди між авторами та видавцями – коли авторські права передаються від авторів до видавця. В такому разі автори втрачають права власності на свій твір та не можуть його використовувати в будь-який спосіб.