Solving applied problems of elasticity theory in geomechanics using the method of argument functions of a complex variable
DOI:
https://doi.org/10.15587/1729-4061.2022.265673Keywords:
soil arrays, soil mechanics, stressed state, argument functions, half-spaceAbstract
When solving many tasks related to mine workings, rock pressure management, development systems, support structures, the issues of strength and stability of rocks become relevant. Limitations and gaps are identified, emphasizing the need for further research and development of new methods for solving applied problems of elasticity theory.
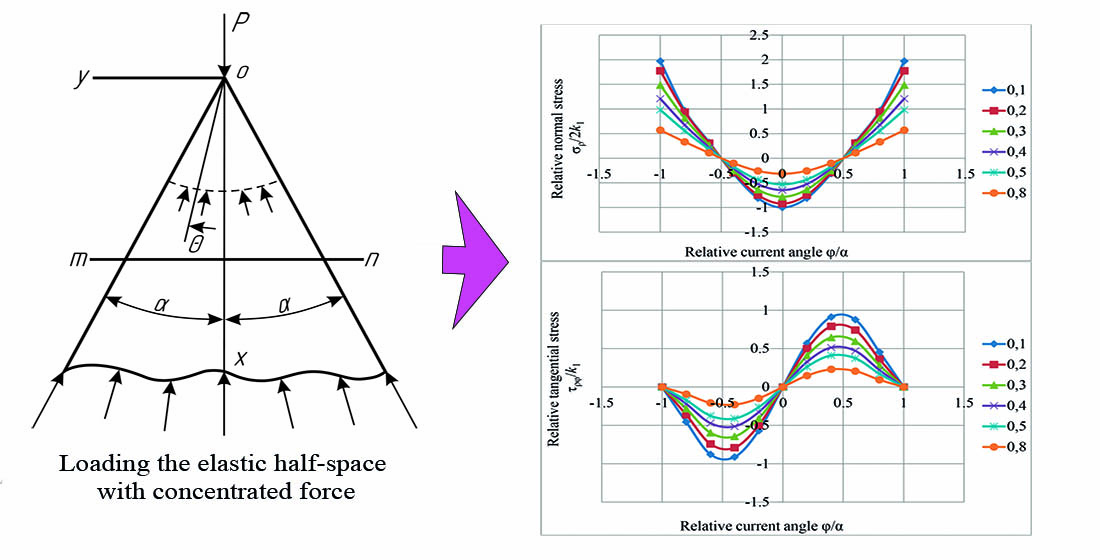
It is of theoretical and practical interest to determine the influence of half-space geometry on the stressed state of the medium and to assess whether it would suffice, in this case, to confine oneself to radial stress when characterizing the stressed state. To build a mathematical model of the stressed state of the array, a complex variable function argument method was used. Based on the developed complex variable function argument method, the applied problem of mechanics on loading the wedge with a concentrated force in polar coordinates was solved.
A feature of the proposed approach is the introduction of tangential stresses with the need to meet boundary conditions along inclined faces. The introduction to the consideration of tangential stress shows that it cannot be neglected at a certain stage of the search for a solution. First of all, this is due to the half-space geometry, the angle at the apex, and the depth of the array. When changing the angle of the wedge, the interface surface changes fundamentally and can pass from a convex shape to a concave one. Simplification of the proposed expressions leads to a complete coincidence with the solutions by other authors obtained by the stress method, which indicates the reliability of the result reported here. This method may be advanced by complicating the half-space geometry, as well as loading, and by building a mathematical model for assessing the effect of tangent stresses on the strength and stability of soils
References
- Loveridge, F., McCartney, J. S., Narsilio, G. A., Sanchez, M. (2020). Energy geostructures: A review of analysis approaches, in situ testing and model scale experiments. Geomechanics for Energy and the Environment, 22, 100173. doi: https://doi.org/10.1016/j.gete.2019.100173
- Kovalevska, I., Samusia, V., Kolosov, D., Snihur, V., Pysmenkova, T. (2020). Stability of the overworked slightly metamorphosed massif around mine working. Mining of Mineral Deposits, 14 (2), 43–52. doi: https://doi.org/10.33271/mining14.02.043
- Dinnik, A. N. (1925). O davlenii gornykh porod i raschete krepi krugloy shakhty. Inzhenernyy rabotnik, 7, 1–12.
- Timoshenko, S. P., Gud'er, Dzh. (1979). Teoriya uprugosti. Moscow: Nauka, 560.
- Nikiforov, S. N. (1955). Teoriya uprugosti i plastichnosti. Moscow: GILSI, 284.
- Bezukhov, N. I. (1968). Osnovy teorii uprugosti, plastichnosti i polzuchesti. Moscow: Vysshaya shkola, 512.
- Bartolomey, A. A. (2004). Mekhanika gruntov. Moscow: Izd-vo ASV, 303.
- Pol'shin, D. E. (1933). Opredelenie napryazheniy v grunte pri nagruzke chasti ego poverkhnosti. Sb. trudov Vsesoyuzn. in-ta osnovan. sooruzh., 1, 39–59.
- Tsytovich, N. A. (1983). Mekhanika gruntov. Moscow: Vysshaya shkola, 216.
- Gersevanov, N. M., Pol'shin, D. E. (1948). Teoreticheskie osnovy mekhaniki gruntov i ikh prakticheskoe primenenie. Moscow: Stroyizdat, 248.
- Yu, J., Yao, W., Duan, K., Liu, X., Zhu, Y. (2020). Experimental study and discrete element method modeling of compression and permeability behaviors of weakly anisotropic sandstones. International Journal of Rock Mechanics and Mining Sciences, 134, 104437. doi: https://doi.org/10.1016/j.ijrmms.2020.104437
- Shen, B., Duan, Y., Luo, X., van de Werken, M., Dlamini, B., Chen, L. et. al. (2020). Monitoring and modelling stress state near major geological structures in an underground coal mine for coal burst assessment. International Journal of Rock Mechanics and Mining Sciences, 129, 104294. doi: https://doi.org/10.1016/j.ijrmms.2020.104294
- Do, D.-P., Tran, N.-H., Dang, H.-L., Hoxha, D. (2019). Closed-form solution of stress state and stability analysis of wellbore in anisotropic permeable rocks. International Journal of Rock Mechanics and Mining Sciences, 113, 11–23. doi: https://doi.org/10.1016/j.ijrmms.2018.11.002
- Tikhonov, A. N., Samarskiy, A. A. (1999). Uravneniya matematicheskoy fiziki. Moscow: Izd-vo MGU, 799.
- Koshlyakov, N. S., Gliner, E. B., Smirnov, M. M. (1970). Uravneniya v chastnykh proizvodnykh matematicheskoy fiziki. Moscow: Vysshaya shkola, 710.
- Vinay, L. S., Bhattacharjee, R. M., Ghosh, N., Budi, G., Kumar, J. V., Kumar, S. (2022). Numerical study of stability of coal pillars under the influence of line of extraction. Geomatics, Natural Hazards and Risk, 13 (1), 1556–1570. doi: https://doi.org/10.1080/19475705.2022.2088409
- Xie, B., Yan, Z., Du, Y., Zhao, Z., Zhang, X. (2019). Determination of Holmquist–Johnson–Cook Constitutive Parameters of Coal: Laboratory Study and Numerical Simulation. Processes, 7 (6), 386. doi: https://doi.org/10.3390/pr7060386
- Wijesinghe, D. R., Dyson, A., You, G., Khandelwal, M., Song, C., Ooi, E. T. (2022). Development of the scaled boundary finite element method for image-based slope stability analysis. Computers and Geotechnics, 143, 104586. doi: https://doi.org/10.1016/j.compgeo.2021.104586
- Sherzer, G. L., Alghalandis, Y. F., Peterson, K., Shah, S. (2022). Comparative study of scale effect in concrete fracturing via Lattice Discrete Particle and Finite Discrete Element Models. Engineering Failure Analysis, 135, 106062. doi: https://doi.org/10.1016/j.engfailanal.2022.106062
- Pariseau, W. G., McCarter, M. K., Wempen, J. M. (2019). Comparison of closure measurements with finite element model results in an underground coal mine in central Utah. International Journal of Mining Science and Technology, 29 (1), 9–15. doi: https://doi.org/10.1016/j.ijmst.2018.11.013
- Sneddon, I. N., Berri, D. S. (1961). Klassicheskaya teoriya uprugosti. Moscow: Gos. izd-vo fiz.-mat. lit-ry, 219.
- Sinekop, N. S., Lobanova, L. S., Parkhomenko, L. A. (2015). Metod R–funktsiy v dinamicheskikh zadachakh teorii uprugosti. Kharkiv, 95.
- Muskhelishvili, N. I. (1966). Nekotorye osnovnye zadachi matematicheskoy teorii uprugosti. Moscow: Nauka, 709.
- Pozharskiy, D. A. (2017). Kontaktnaya zadacha dlya ortotropnogo poluprostranstva. Mekhanika tverdogo tela, 3, 100–108.
- Cassiani, G., Brovelli, A., Hueckel, T. (2017). A strain-rate-dependent modified Cam-Clay model for the simulation of soil/rock compaction. Geomechanics for Energy and the Environment, 11, 42–51. doi: https://doi.org/10.1016/j.gete.2017.07.001
- Vasiliev, L. M., Vasiliev, D. L., Malich, M. G., Anhelovskyi, O. O. (2017). Analytical method for calculating and charting “stress–deformation” provided longitudinal form of destruction of rock samples. Naukovyi Visnyk Natsionalnoho Hirnychoho Universytetu, 3, 68–74. Available at: http://www.nvngu.in.ua/index.php/en/component/jdownloads/finish/68-03/8659-03-2017-vasiliev/0
- Chigirinsky, V., Putnoki, A. (2017). Development of a dynamic model of transients in mechanical systems using argument-functions. Eastern-European Journal of Enterprise Technologies, 3 (7 (87)), 11–22. doi: https://doi.org/10.15587/1729-4061.2017.101282
- Chigirinsky, V., Naumenko, O. (2019). Studying the stressed state of elastic medium using the argument functions of a complex variable. Eastern-European Journal of Enterprise Technologies, 5 (7 (101)), 27–35. doi: https://doi.org/10.15587/1729-4061.2019.177514
- Chigirinsky, V., Naumenko, O. (2020). Invariant differential generalizations in problems of the elasticity theory as applied to polar coordinates. Eastern-European Journal of Enterprise Technologies, 5 (7 (107)), 56–73. doi: https://doi.org/10.15587/1729-4061.2020.213476
- Chigirinsky, V., Naizabekov, A., Lezhnev, S. (2021). Closed problem of plasticity theory. Journal of Chemical Technology and Metallurgy, 56 (4), 867–876.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2022 Valeriy Chigirinsky, Abdrakhman Naizabekov, Sergey Lezhnev, Sergey Kuzmin, Olena Naumenko

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.
A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.








