Identification of the patterns of influence the number of reinforcing elements and the inhomogeneity parameter of the shell material on frequencies of a reinforced inhomogeneous orthotropic spherical shell with a medium
DOI:
https://doi.org/10.15587/1729-4061.2022.266166Keywords:
spherical shell, free oscillation, frequency, Legendre polynomial, spherical Bessel functionsAbstract
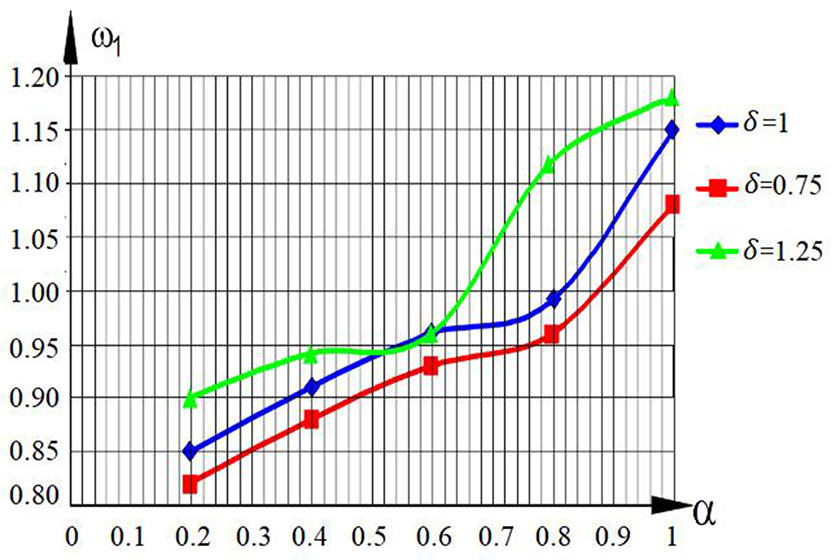
Spherical shells are used in many areas of the national economy. Spherical domes are widely used in the construction of various structures (technoparks, testing laboratories, entertainment complexes, reservoirs, etc.). They are also used in aircraft, ship structures, radar antennas and other structures. It is known that coatings have sufficient strength and durability even with a small thickness. However, to increase the working life of coatings, to ensure their long-term operation, as well as to increase their hardness, it is necessary to strengthen them on the surface or inside with rods. Sometimes it is possible to reduce the weight of the structure and save material consumption by strengthening it with. One of the advantages of these structures is that they give the maximum useful volume, being both load-bearing and enclosing structures. Checking the shells for stability is a priority task, since it is known that the shells, even with an insignificant thickness, have great strength and therefore their insufficient stability can be a criterion determining the bearing capacity. This article is devoted to identifying the regularities of the influence of the number of reinforcing elements and the inhomogeneity parameter of the shell material on the frequencies supported by an inhomogeneous orthotropic spherical shell with a medium. To solve the problem under consideration, the Hamilton-Ostrogradsky variation principle is applied. The frequency equation is constructed and implemented numerically. Such studies have not been considered for a reinforced spherical shell with a no uniform filler in thickness
Supporting Agency
- We express our gratitude to the Professor of the Department of Mechanics of the Azerbaijan University of Architecture and Construction, Doctor of Mathematical Sciences, R.A. Iskandarov for his help in constructing a computational model of free oscillations of a reinforced inhomogeneous spherical shell with a filler.
References
- Latifov, F. S. (1988). Asymptotic analysis of the problem of free vibrations of a spherical shell with viscous elastic filler. In: Mechanics of deformable solids. Baku: Elm, 159–167.
- Latifov, F. S., Agayev, R. N. (2018). Oscillations of Longitudinally Reinforced Heterogeneous Orthotropic Cylindrical Shell with Flowing Liquid. International Journal on Technical and Physical Problems of Engineering (IJTPE), 10 (34), 41–45. Available at: https://www.iotpe.com/IJTPE/IJTPE-2018/IJTPE-Issue34-Vol10-No1-Mar2018/7-IJTPE-Issue34-Vol10-No1-Mar2018-pp41-45.pdf
- Agayev, R. (2020). Vibrations of an inhomogeneous cylindrical shell dynamically interacting with motive fluid and stiffened with rings. International Journal on Technical and Physical Problems of Engineering (IJTPE), 12 (42), 31–34. Available at: https://www.iotpe.com/IJTPE/IJTPE-2020/IJTPE-Issue42-Vol12-No1-Mar2020/6-IJTPE-Issue42-Vol12-No1-Mar2020-pp31-34.pdf
- Agayev, R. N. (2020). Vibrations of a heterogeneous cylindrical shell stiffened with rings and dynamically contacting with flowing fluid. International Journal on Technical and Physical Problems of Engineering (IJTPE), 12 (43), 83–87. Available at: https://www.iotpe.com/IJTPE/IJTPE-2020/IJTPE-Issue43-Vol12-No2-Jun2020/15-IJTPE-Issue43-Vol12-No2-Jun2020-pp83-87.pdf
- Iskanderov, R. A., Tabatabaei, J. M. (2020). Vibrations of fluid-filled inhomogeneous cylindrical shells strengthened with lateral ribs. International Journal on Technical and Physical Problems of Engineering (IJTPE), 12 (42), 121–125. Available at: https://www.iotpe.com/IJTPE/IJTPE-2020/IJTPE-Issue42-Vol12-No1-Mar2020/23-IJTPE-Issue42-Vol12-No1-Mar2020-pp121-125.pdf
- Tabatabaei, J. M. (2022). Vibrations of longitudinally stiffened elastic medium- contacting cylindrical sheels. Tran. Natl. Acad. Sci. Azerb. Ser. Phys.-Tech. Math. Sci. Mechanics, 42 (7), 23–42.
- Suleimenov, U., Zhangabay, N., Utelbayeva, A., Azmi Murad, M. A., Dosmakanbetova, A., Abshenov, K. et al. (2022). Estimation of the strength of vertical cylindrical liquid storage tanks with dents in the wall. Eastern-European Journal of Enterprise Technologies, 1 (7 (115)), 6–20. doi: https://doi.org/10.15587/1729-4061.2022.252599
- Fomin, O., Lovska, A., Khara, M., Nikolaienko, I., Lytvynenko, A., Sova, S. (2022). Adapting the load-bearing structure of a gondola car for transporting high-temperature cargoes. Eastern-European Journal of Enterprise Technologies, 2 (7 (116)), 6–13. doi: https://doi.org/10.15587/1729-4061.2022.253770
- Kovalchuk, V., Sobolevska, Y., Onyshchenko, A., Bal, O., Kravets, I., Pentsak, A. et al. (2022). Investigating the influence of the diameter of a fiberglass pipe on the deformed state of railroad transportation structure “embankment-pipe.” Eastern-European Journal of Enterprise Technologies, 2 (7 (116)), 35–43. doi: https://doi.org/10.15587/1729-4061.2022.254573
- Gameliak, I., Dmytrychenko, A., Tsybulskyi, V., Kharchenko, A. (2022). Determining the effect of reinforcing a cement-concrete coating of bridges on the stressed-strained state of structures. Eastern-European Journal of Enterprise Technologies, 1 (7 (115)), 21–31. doi: https://doi.org/10.15587/1729-4061.2022.251189
- Akhundov, V. M. (2020). Method for Calculating the Near-Surface Effect in Piecewise Homogeneous Bodies at Large Deformations Based on a Two-Level Approach. Mechanics of Composite Materials, 56 (2), 169–184. doi: https://doi.org/10.1007/s11029-020-09870-w
- Amiro, I. Ya., Zarutskii, V. A. (1980). Theory of ribbed shells. Methods for Calculating Shells. Kyiv, 367.
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2022 Etibar Guliyev, Rashad Allahverdiyev, Qezale Kheyrabadi

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.
A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.








