Computer analysis of multiple measurements with the sensor's quadratic transformation function

Authors

DOI:

https://doi.org/10.15587/1729-4061.2023.273299

Keywords:

redundancy, multiple measurements, quadratic transformation function, function parameters, accuracy improvement

Abstract

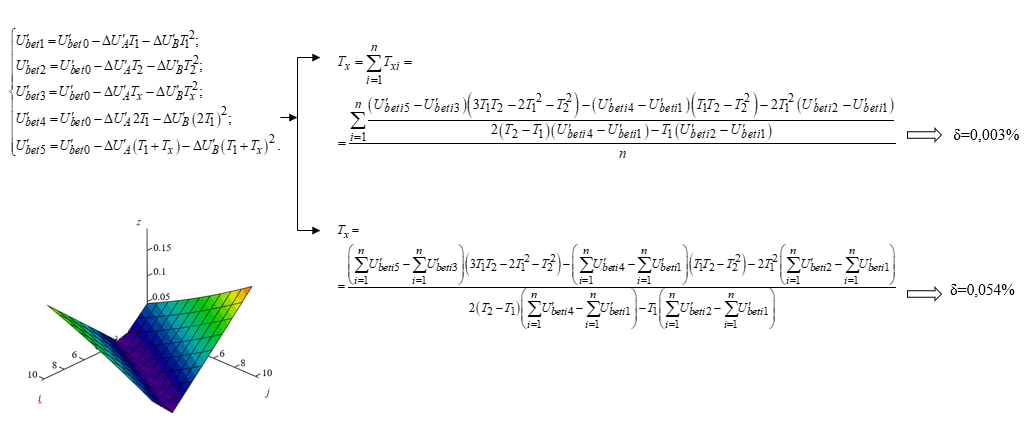
The object of research is multiple measurements. The research aims to improve the accuracy of multiple measurements with a non-linear and unstable sensor transformation function. It is proved that the redundant measurement equation ensures the independence of the measurement result from the parameters of the transformation function and their deviations from the nominal values. It was found that the result of redundant measurements is affected by the reproduction errors of normalized temperatures T1 and T2. It is shown that the best accuracy results are obtained with a reproduction error of normalized temperature T2 within ±1.0 % and temperature T1 within ±0.1 %. This makes it possible to reduce the accuracy requirements for the source of reproduction of normalized temperature Т2.

The possibility of processing the results of multiple measurements by two approaches is presented. Computer modeling using the first approach found that with a reproduction error of normalized temperature T2 within ±0.5 %, the relative measurement error is 0.003 %. When modeling the second approach, the relative error is 0.05 %. It was also found that with an increase in the reproduction error of normalized temperature T2 to ±1.0 %, the value of the relative error is 0.04 %. Due to this, when applying the second approach, it becomes possible to choose a non-high-precision source of reproduction of normalized temperature T2. In addition, the sensitivity of the second approach to the digit range of measuring devices was found, which leads to the dependence of the measurement result on their accuracy.

There are reasons to assert the possibility of increasing the accuracy of multiple measurements by processing the results of intermediate measurements according to redundant measurement equations using two approaches

Author Biographies

Volodymyr Shcherban’, Kyiv National University of Technologies and Design

Doctor of Technical Sciences, Professor, Head of Department

Department of Computer Science

Hanna Korohod, Kyiv National University of Technologies and Design

PhD, Associate Professor

Department of Computer Science

Nataliia Chuprynka, Kyiv National University of Technologies and Design

PhD

Department of Computer Science

Oksana Kolysko, Kyiv National University of Technologies and Design

PhD, Associate Professor

Department of Computer Science

Yury Shcherban’, State Higher Educational Establishment "Kyiv College of Light Industry"

Doctor of Technical Sciences, Professor, Head of Department

Department of Light Industry Technologies

Ganna Shchutska, State Higher Educational Establishment "Kyiv College of Light Industry"

Doctor of Technical Sciences, Associate Professor

Director

Department of Light Industry Technologies

References

  1. Rishan, O. Y., Matviienko, N. V. (2014). Strukturni metody pidvyshchennia tochnosti vymiriuvan v avtomatychnykh systemakh dozuvannia sypkykh materialiv z vykorystanniam mahnitopruzhnykh pervynnykh vymiriuvalnykh peretvoriuvachiv zusyllia. Naukovo-tekhnichna informatsiya, 4, 47–51. Available at: http://nbuv.gov.ua/UJRN/NTI_2014_4_11
  2. Shcherban’, V., Kolysko, O., Melnyk, G., Sholudko, M., Shcherban’, Y. Shchutska, G. (2020). Determining tension of yarns when interacting with guides and operative parts of textile machinery having the torus form. Fibres and Textiles, 4, 87–95. Available at: http://vat.ft.tul.cz/2020/4/VaT_2020_4_12.pdf
  3. Vdovichenko, A., Tuz, J. (2018). Accuracy enhancement of active power measurement with significant reactive load by creation of the shunt middle point. Measuring Equipment and Metrology, 79 (1), 76–81. doi: https://doi.org/10.23939/istcmtm2018.01.076
  4. Horbatyi, I. V. (2017). Improving measuring accuracy of inharmonious signal voltage under the additive noise condition. Tehnologiya i konstruirovanie v elektronnoy apparature, 1-2, 7–15. doi: https://doi.org/10.15222/tkea2017.1-2.07
  5. Pan, D., Jiang, Z., Gui, W., Yang, C., Xie, Y., Jiang, K. (2018). A method for improving the accuracy of infrared thermometry under the influence of dust. IFAC-PapersOnLine, 51 (21), 246–250. doi: https://doi.org/10.1016/j.ifacol.2018.09.426
  6. Zhang, Y., Chen, Y., Fu, X., Luo, C. (2016). A method for reducing the influence of measuring distance on infrared thermal imager temperature measurement accuracy. Applied Thermal Engineering, 100, 1095–1101. doi: https://doi.org/10.1016/j.applthermaleng.2016.02.119
  7. Lewis, G., Merken, P., Vandewal, M. (2018). Enhanced Accuracy of CMOS Smart Temperature Sensors by Nonlinear Curvature Correction. Sensors, 18 (12), 4087. doi: https://doi.org/10.3390/s18124087
  8. Koritsoglou, K., Christou, V., Ntritsos, G., Tsoumanis, G., Tsipouras, M. G., Giannakeas, N., Tzallas, A. T. (2020). Improving the Accuracy of Low-Cost Sensor Measurements for Freezer Automation. Sensors, 20 (21), 6389. doi: https://doi.org/10.3390/s20216389
  9. Koestoer, R. A., Saleh, Y. A., Roihan, I., Harinaldi (2019). A simple method for calibration of temperature sensor DS18B20 waterproof in oil bath based on Arduino data acquisition system. AIP Conference Proceedings. doi: https://doi.org/10.1063/1.5086553
  10. Chen, C.-C., Chen, C.-L., Lin, Y. (2016). All-Digital Time-Domain CMOS Smart Temperature Sensor with On-Chip Linearity Enhancement. Sensors, 16 (2), 176. doi: https://doi.org/10.3390/s16020176
  11. Su, J., Roshchupkina, N., Kochan, V., Roshchupkin, O., Sachenko, A. (2016). Methods for improving the accuracy of sensors with a significant influence of non-informative factors. 2016 IEEE Sensors Applications Symposium (SAS). doi: https://doi.org/10.1109/sas.2016.7479894
  12. Dorozinska, H. V. (2020). Evaluation Numerical Methods Effectiveness for Processing of Measurement Results by Improved SPR-Sensor. Visnyk of Vinnytsia Politechnical Institute, 2, 7–13. doi: https://doi.org/10.31649/1997-9266-2020-149-2-7-13
  13. Bedenik, G., Souza, M., Carvalho, E. A. N., Molina, L., Montalvao, J., Freire, R. (2022). Analysis of Parameters Influence in a MOX Gas Sensor Model. 2022 IEEE International Instrumentation and Measurement Technology Conference (I2MTC). doi: https://doi.org/10.1109/i2mtc48687.2022.9806695
  14. Kondratov, V. T. (2014). The problems solved by methods of redundant measurements. Vymiriuvalna ta obchysliuvalna tekhnika v tekhnolohichnykh protsesakh – 2014 (VOTTP-14 2014). Odessa, 26–30. Available at: https://biblio.suitt.edu.ua/bitstream/handle/123456789/1990/vottp-2014-konf.pdf?sequence=1&isAllowed=y
  15. Kondratov, V. T. (2010). Metody izbytochnyh izmereniy: osnovnye opredeleniya i klassifikaciya. Visnyk Khmelnytskoho natsionalnoho universytetu, 3, 220–232. Available at: http://journals.khnu.km.ua/vestnik/pdf/tech/2010_3/47kon.pdf
  16. Kondratov, V. T. (2009). Teoriya izbytochnyh izmereniy: universal'noe uravnenie izmereniy. Visnyk Khmelnytskoho natsionalnoho universytetu, 5, 116–129. Available at: http://journals.khnu.km.ua/vestnik/pdf/tech/2009_5/zmist.files/23kon.pdf
  17. Shcherban, V., Korogod, G., Chaban, V., Kolysko, O., Shcherban’, Y., Shchutska, G. (2019). Computer simulation methods of redundant measurements with the nonlinear transformation function. Eastern-European Journal of Enterprise Technologies, 2 (5 (98)), 16–22. doi: https://doi.org/10.15587/1729-4061.2019.160830
  18. Shcherban’, V., Korogod, G., Kolysko, O., Kolysko, M., Shcherban’, Y., Shchutska, G. (2020). Computer simulation of multiple measurements of logarithmic transformation function by two approaches. Eastern-European Journal of Enterprise Technologies, 6 (4 (108)), 6–13. doi: https://doi.org/10.15587/1729-4061.2020.218517
  19. Shcherban’, V., Korogod, G., Kolysko, O., Kolysko, M., Shcherban’, Y., Shchutska, G. (2021). Computer simulation of logarithmic transformation function to expand the range of high-precision measurements. Eastern-European Journal of Enterprise Technologies, 2 (9 (110)), 27–36. doi: https://doi.org/10.15587/1729-4061.2021.227984
  20. Shcherban’, V., Korogod, G., Kolysko, O., Volivach, A., Shcherban’, Y., Shchutska, G. (2022). Computer modeling in the study of the effect of normalized quantities on the measurement accuracy of the quadratic transformation function. Eastern-European Journal of Enterprise Technologies, 2 (5 (116)), 6–16. doi: https://doi.org/10.15587/1729-4061.2022.254337
  21. Boyko, O., Barylo, G., Holyaka, R., Hotra, Z., Ilkanych, K. (2018). Development of signal converter of thermal sensors based on combination of thermal and capacity research methods. Eastern-European Journal of Enterprise Technologies, 4 (9 (94)), 36–42. doi: https://doi.org/10.15587/1729-4061.2018.139763
Computer analysis of multiple measurements with the sensor's quadratic transformation function

Downloads

Published

2023-02-28

How to Cite

Shcherban’, V., Korohod, H., Chuprynka, N., Kolysko, O., Shcherban’, Y., & Shchutska, G. (2023). Computer analysis of multiple measurements with the sensor’s quadratic transformation function. Eastern-European Journal of Enterprise Technologies, 1(5 (121), 17–25. https://doi.org/10.15587/1729-4061.2023.273299

Issue

Vol. 1 No. 5 (121) (2023): Applied physics

Section

Applied physics

License

Copyright (c) 2023 Volodymyr Shcherban’, Hanna Korohod, Nataliia Chuprynka, Oksana Kolysko, Yury Shcherban’, Ganna Shchutska

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.

A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.