Approach to the synthesis of an aperiodic robust automatic control system based on the gradient-speed method of Lyapunov vector functions
DOI:
https://doi.org/10.15587/1729-4061.2023.274063Keywords:
robust stability, linear multidimensional systems, Lyapunov vector-functions, aperiodic robustly stable systemsAbstract
One of the actual problems for the theory and practice of control of dynamic objects is the development of methods for research and synthesis of control systems of multidimensional objects.
The paper proposes a universal approach to construct Lyapunov vector functions directly from the equation of state of control system and a new gradient-speed method of Lyapunov vector functions to study aperiodic robust stability of linear control system with m inputs and n outputs.
The study of aperiodic robust stability of automatic control systems is based on the construction of Lyapunov vector functions and gradient-speed dynamic control systems.
The basic statements of Lyapunov's theorem about asymptotic stability and notions of stability of dynamic systems are used. The representation of control systems as gradient systems and Lyapunov functions as potential functions of gradient systems from the catastrophe theory allow to construct the full-time derivative of Lyapunov vector functions always as a sign-negative function equal to the scalar product of the velocity vector on the gradient vector. The conditions of aperiodic robust stability are obtained as a system of inequalities on the uncertain parameters of the automatic control system, which are a condition for the existence of the Lyapunov vector-function.
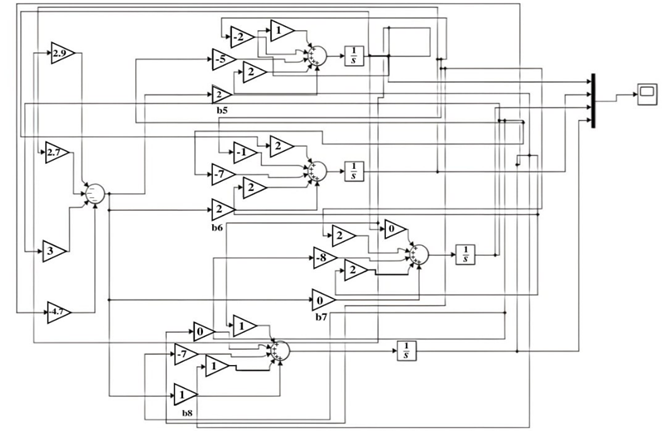
A numerical example of synthesis of aperiodic robustness of a multidimensional control object is given. The example shows the main stages of the developed synthesis method, the study of the system stability at different values of the coefficients k, confirming the consistency of the proposed method. Transients in the system satisfy all requirements
References
- Beisenbi, M., Kaliyeva, S., Sagymbay, A., Abdugulova, Z., Ostayeva, A. (2021). A new approach for synthesis of the control system by gradient-velocity method of Lyapunov vector functions. Journal of Theoretical and Applied Information Technology, 2 (99), 381–389. Available at: http://www.jatit.org/volumes/Vol99No2/11Vol99No2.pdf
- Chen, H.-G., Han, K.-W. (1997). Robust Controller Design with Damping and Stability Specifications. Journal of Guidance, Control, and Dynamics, 20 (2), 400–403. doi: https://doi.org/10.2514/2.4055
- Chen, H.-G., Han, K.-W. (1993). Stability-robustness analysis for linear systems with state-space models. Journal of the Franklin Institute, 330 (5), 939–966. doi: https://doi.org/10.1016/0016-0032(93)90087-b
- Barbashin, E. A. (1967). Introduction in the theory of stability. Moscow: Nauka.
- Andreev, Y. N. (1976). Control of finite dimensional linear objects. Moscow: Nauka.
- Bailey, F. N. (1965). The Application of Lyapunov’s Second Method to Interconnected Systems. Journal of the Society for Industrial and Applied Mathematics Series A Control, 3 (3), 443–462. doi: https://doi.org/10.1137/0303030
- Beisenbi, M., Uskenbayeva, G. (2014). The New Approach of Design Robust Stability for Linear Control System. Proceedings of the International Conference on Advances in Electronics and Electrical Technology, Institute of Research engineers and Doctors. Bangkok, 11–18.
- Kuznetsov, N. V. (2020). Theory of Hidden Oscillations and Stability of Control Systems. Journal of Computer and Systems Sciences International, 59 (5), 647–668. doi: https://doi.org/10.1134/s1064230720050093
- Petridis, V., Petridis, S. (2006). Construction of Neural Network Based Lyapunov Functions. The 2006 IEEE International Joint Conference on Neural Network Proceedings. doi: https://doi.org/10.1109/ijcnn.2006.247233
- Parkhomenko, L., Butko, T., Prokhorov, V., Kalashnikova, T., Golovko, T. (2022). Building a model for planning rapid delivery of containers by rail under the conditions of intermodal transportation based on robust optimization. Eastern-European Journal of Enterprise Technologies, 5 (3 (119)), 6–16. doi: https://doi.org/10.15587/1729-4061.2022.265668
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2023 Мamyrbek Beisenbi, Samal Kaliyeva

This work is licensed under a Creative Commons Attribution 4.0 International License.
The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.
A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.








