Розробка нового легковагового алгоритму шифрування

Автор(и)

DOI:

https://doi.org/10.15587/1729-4061.2023.280055

Ключові слова:

алгоритм шифрування, легковаговий алгоритм, криптографічні перетворення, лавинний ефект, криптостійкість

Анотація

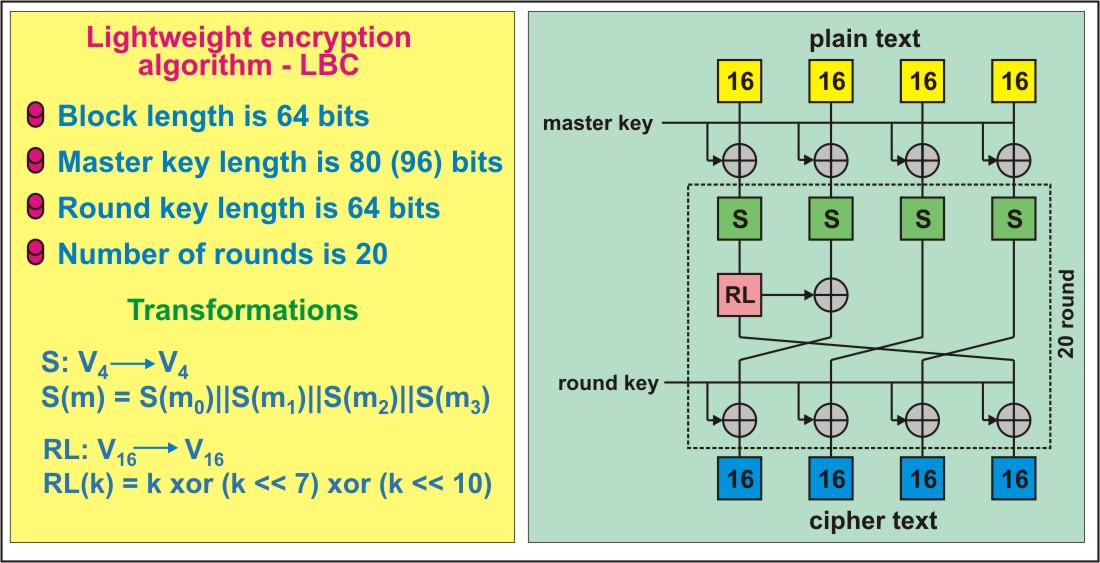
Алгоритми легковагового шифрування вважаються відносно новим напрямом у розвитку криптографії із закритим ключем. Така потреба виникла внаслідок появи великої кількості пристроїв з невеликою обчислювальною потужністю та пам'яттю. Тому з’явилась необхідність розробки алгоритмів, здатних забезпечити достатній рівень безпеки, при мінімальному використанні ресурсів. У роботі представлено новий легковаговий алгоритм шифрування LBC. LBC – це 64-бітовий симетричний блоковий алгоритм. Він підтримує 80 битий секретний ключ. Кількість раундів – 20. Алгоритм має структуру мережі Фейстеля. Розроблений легковаговий алгоритм має просту схему реалізації, а перетворення, що використовуються в даному алгоритмі, мають добрі криптографічні властивості. Це перевірено при дослідженні криптографічних властивостей алгоритму за допомогою «лавинного ефекту» та статистичних тестів. Перевірка лавинної властивості виконувалася кожного раунду при зміні кожного біта вихідного тексту. На підставі проведених робіт встановлено, що запропонований алгоритм шифрування ефективний для забезпечення гарного лавинного ефекту та бінарна послідовність, отримана після зашифрування, близька до випадкової. Також оцінено його захищеність від лінійного та диференціального криптоаналізу. Результати досліджень виявили добрі криптографічні властивості даного алгоритму. Алгоритм буде застосовуватися для пристроїв, що володіють малими апаратними ресурсами, в інформаційно-комунікаційних системах, де циркулюють відомості конфіденційного характеру, а також при необхідності в оперативно прийнятні терміни обмінюватися інформацією в захищеному вигляді

Біографії авторів

Nursulu Kapalova, Institute of Information and Computational Technologies

Leading Researcher, Candidate of Technical Sciences, Associate Professor

Information Security Laboratory

Kunbolat Algazy, Institute of Information and Computational Technologies

Senior Researcher, PhD

Information Security Laboratory

Armanbek Haumen, Institute of Information and Computational Technologies

Research Associate

Information Security Laboratory

Посилання

  1. Usman, M., Ahmed, I., Imran, M., Khan, S., Ali, U. (2017). SIT: A Lightweight Encryption Algorithm for Secure Internet of Things. International Journal of Advanced Computer Science and Applications, 8 (1). doi: https://doi.org/10.14569/ijacsa.2017.080151
  2. Yun, J., Kim, M. (2020). JLVEA: Lightweight Real-Time Video Stream Encryption Algorithm for Internet of Things. Sensors, 20 (13), 3627. doi: https://doi.org/10.3390/s20133627
  3. Taresh, H. (2018). LT10 a lightweight proposed encryption algorithm for IOT. Iraqi Journal for Computers and Informatics, 44 (1), 1–5. doi: https://doi.org/10.25195/ijci.v44i1.64
  4. Beaulieu, R., Shors, D., Smith, J., Treatman-Clark, S., Weeks, B., Wingers, L. (2013). Paper 2013/404. The SIMON and SPECK families of lightweight block ciphers. Cryptology ePrint Archive. Available at: https://eprint.iacr.org/2013/404
  5. Gu, D., Li, J., Li, S., Ma, Z., Guo, Z., Liu, J. (2012). Differential Fault Analysis on Lightweight Blockciphers with Statistical Cryptanalysis Techniques. 2012 Workshop on Fault Diagnosis and Tolerance in Cryptography. doi: https://doi.org/10.1109/fdtc.2012.16
  6. Kumar V G, K., Rai C, S. (2021). Design and Implementation of Novel BRISI Lightweight Cipher for Resource Constrained Devices. Microprocessors and Microsystems, 84, 104267. doi: https://doi.org/10.1016/j.micpro.2021.104267
  7. Yang, W., Wang, R., Guan, Z., Wu, L., Du, X., Guizani, M. (2020). A Lightweight Attribute Based Encryption Scheme with Constant Size Ciphertext for Internet of Things. ICC 2020 - 2020 IEEE International Conference on Communications (ICC). doi: https://doi.org/10.1109/icc40277.2020.9149294
  8. Kazlauskas, K., Kazlauskas, J. (2009). Key-Dependent S-Box Generation in AES Block Cipher System. Informatica, 20 (1), 23–34. doi: https://doi.org/10.15388/informatica.2009.235
  9. Preneel, B. (2010). Perspectives on Lightweight Cryptography. Shanghai. Available at: https://homes.esat.kuleuven.be/~preneel/preneel_lightweight_shanghaiv1.pdf
  10. Ivanov, G., Nikolov, N., Nikova, S. (2016). Cryptographically Strong S-Boxes Generated by Modified Immune Algorithm. Lecture Notes in Computer Science, 31–42. doi: https://doi.org/10.1007/978-3-319-29172-7_3
  11. Horbenko, I. D., Horbenko, Yu. I. (2012). Prykladna kryptolohiya. Teoriya. Praktyka. Zastosuvannia. Kharkiv: Vydavnytstvo «Fort», 870.
  12. Dey, S., Ghosh, R. (2018). A Review of Existing 4-Bit Crypto S-Box Cryptanalysis Techniques and Two New Techniques with 4-Bit Boolean Functions for Cryptanalysis of 4-Bit Crypto S-Boxes*. Advances in Pure Mathematics, 08 (03), 272–306. doi: https://doi.org/10.4236/apm.2018.83015
  13. Khompysh, A., Kapalova, N., Algazy, K., Dyusenbayev, D., Sakan, K. (2022). Design of substitution nodes (S-Boxes) of a block cipher intended for preliminary encryption of confidential information. Cogent Engineering, 9 (1). doi: https://doi.org/10.1080/23311916.2022.2080623
  14. Kapalova, N. A., Khaumen, A., Saқan, K. (2020). Rasseivayuschie svoystva lineynykh preobrazovaniy. Mater. nauch. konf. IIVT MON RK «Sovremennye problemy informatiki i vychislitel'nykh tekhnologiy». Almaty, 191–196. Available at: https://conf.iict.kz/wp-content/uploads/2020/10/mpcsct-collection-08.07.2020-final.pdf
  15. Lisitskaya, I. V., Nastenko, A. A. (2011). Great ciphers - casual substitution. Radiotekhnika, 166, 50–55. Available at: https://openarchive.nure.ua/bitstream/document/15255/1/Radiotehnika_V166_2011_rus.pdf
  16. Teh, J. S., Tham, L. J., Jamil, N., Yap, W.-S. (2022). New differential cryptanalysis results for the lightweight block cipher BORON. Journal of Information Security and Applications, 66, 103129. doi: https://doi.org/10.1016/j.jisa.2022.103129
  17. Biham, E., Shamir, A. (1991). Differential Cryptanalysis of DES-like Cryptosystems. Lecture Notes in Computer Science, 2–21. doi: https://doi.org/10.1007/3-540-38424-3_1
  18. Carlet, C. (2010). Vectorial Boolean Functions for Cryptography. Boolean Models and Methods in Mathematics, Computer Science, and Engineering, 398–470. doi: https://doi.org/10.1017/cbo9780511780448.012
  19. Kim, J., Hong, S., Lim, J. (2010). Impossible differential cryptanalysis using matrix method. Discrete Mathematics, 310 (5), 988–1002. doi: https://doi.org/10.1016/j.disc.2009.10.019
  20. Liu, Y., Liang, H., Wang, W., Wang, M. (2017). New Linear Cryptanalysis of Chinese Commercial Block Cipher Standard SM4. Security and Communication Networks, 2017, 1–10. doi: https://doi.org/10.1155/2017/1461520
  21. Matsui, M. (1994). Linear Cryptanalysis Method for DES Cipher. Lecture Notes in Computer Science, 386–397. doi: https://doi.org/10.1007/3-540-48285-7_33
  22. Liu, Z. (2021). Differential-linear cryptanalysis of PRINCE cipher. Chinese Journal of Network and Information Security, 7 (4), 131–140. doi: https://doi.org/10.11959/j.issn.2096-109x.2021072
  23. Biryukov, A., De Cannière, C. (2011). Linear Cryptanalysis for Block Ciphers. Encyclopedia of Cryptography and Security, 722–725. doi: https://doi.org/10.1007/978-1-4419-5906-5_589
  24. Borghoff, J. (2011). 4.6 Linear Cryptanalysis. Cryptanalysis of Lightweight Ciphers. Technical University of Denmark, 60–65. Available at: https://backend.orbit.dtu.dk/ws/portalfiles/portal/5456432/phd-thesis_Julia_Borghoff.pdf
  25. Vergili, I., Yücel, M. D. (2001). Avalanche and Bit Independence Properties for the Ensembles of Randomly Cho-sen S-Boxes. Turkish Journal of Electrical Engineering and Computer Sciences, 9 (2), 137–146. Available at: https://journals.tubitak.gov.tr/elektrik/vol9/iss2/3
  26. Shnayer, B. (2002). Prikladnaya kriptografiya. Moscow:Triumf, 816.
  27. Babenko, L. K., Ischukova, E. A. (2006). Sovremennye algoritmy blochnogo shifrovaniya i metody ikh analiza. Moscow: «Gelios ARV», 376.
  28. Algazy, K. T., Babenko, L. K., Biyashev, R. G., Ishchukova, E. A., Kapalova, N. A., Nysynbaeva, S. E., Smolarz, A. (2020). Differential Cryptanalysis of New Qamal Encryption Algorithm. International journal of electronics and telecommunications, 66 (4), 647–653. doi: https://doi.org/10.24425/ijet.2020.134023
  29. O’Connor, L. (1995). Properties of linear approximation tables. Lecture Notes in Computer Science, 131–136. doi: https://doi.org/10.1007/3-540-60590-8_10
  30. Kuznetsov, A. A., Lisitskaya, I. V., Isaev, S. A. (2011). Lineynye svoystva blochnykh simmetrichnykh shifrov, predstavlennykh na ukrainskiy konkurs. Prikladnaya radioelektronika, 10 (2), 135–140.
  31. Heys, H. M. (2002). A tutorial on linear and differential cryptanalysis. Cryptologia, 26 (3), 189–221. doi: https://doi.org/10.1080/0161-110291890885
  32. Kapalova, N., Sakan, K., Algazy, K., Dyusenbayev, D. (2022). Development and Study of an Encryption Algorithm. Computation, 10 (11), 198. doi: https://doi.org/10.3390/computation10110198
  33. Bogdanov, A., Knudsen, L. R., Leander, G., Paar, C., Poschmann, A., Robshaw, M. J. B. et al. (2007). PRESENT: An Ultra-Lightweight Block Cipher. Lecture Notes in Computer Science, 450–466. doi: https://doi.org/10.1007/978-3-540-74735-2_31
Розробка нового легковагового алгоритму шифрування

##submission.downloads##

Опубліковано

2023-06-30

Як цитувати

Kapalova, N., Algazy, K., & Haumen, A. (2023). Розробка нового легковагового алгоритму шифрування. Eastern-European Journal of Enterprise Technologies, 3(9 (123), 6–19. https://doi.org/10.15587/1729-4061.2023.280055

Номер

Том 3 № 9 (123) (2023): Інформаційно-керуючі системи

Розділ

Інформаційно-керуючі системи

Ліцензія

Авторське право (c) 2023 Nursulu Kapalova, Kunbolat Algazy, Armanbek Haumen

Creative Commons License

Ця робота ліцензується відповідно до Creative Commons Attribution 4.0 International License.

Закріплення та умови передачі авторських прав (ідентифікація авторства) здійснюється у Ліцензійному договорі. Зокрема, автори залишають за собою право на авторство свого рукопису та передають журналу право першої публікації цієї роботи на умовах ліцензії Creative Commons CC BY. При цьому вони мають право укладати самостійно додаткові угоди, що стосуються неексклюзивного поширення роботи у тому вигляді, в якому вона була опублікована цим журналом, але за умови збереження посилання на першу публікацію статті в цьому журналі.

Ліцензійний договір – це документ, в якому автор гарантує, що володіє усіма авторськими правами на твір (рукопис, статтю, тощо).
Автори, підписуючи Ліцензійний договір з ПП «ТЕХНОЛОГІЧНИЙ ЦЕНТР», мають усі права на подальше використання свого твору за умови посилання на наше видання, в якому твір опублікований. Відповідно до умов Ліцензійного договору, Видавець ПП «ТЕХНОЛОГІЧНИЙ ЦЕНТР» не забирає ваші авторські права та отримує від авторів дозвіл на використання та розповсюдження публікації через світові наукові ресурси (власні електронні ресурси, наукометричні бази даних, репозитарії, бібліотеки тощо).
За відсутності підписаного Ліцензійного договору або за відсутністю вказаних в цьому договорі ідентифікаторів, що дають змогу ідентифікувати особу автора, редакція не має права працювати з рукописом.
Важливо пам’ятати, що існує і інший тип угоди між авторами та видавцями – коли авторські права передаються від авторів до видавця. В такому разі автори втрачають права власності на свій твір та не можуть його використовувати в будь-який спосіб.