Topology optimization for isotropic elastic materials using honeycomb tessell

Authors

DOI:

https://doi.org/10.15587/1729-4061.2023.277909

Keywords:

topology optimization, boundary conditions, isotropic material, filter sensitivity, honeycomb tessell

Abstract

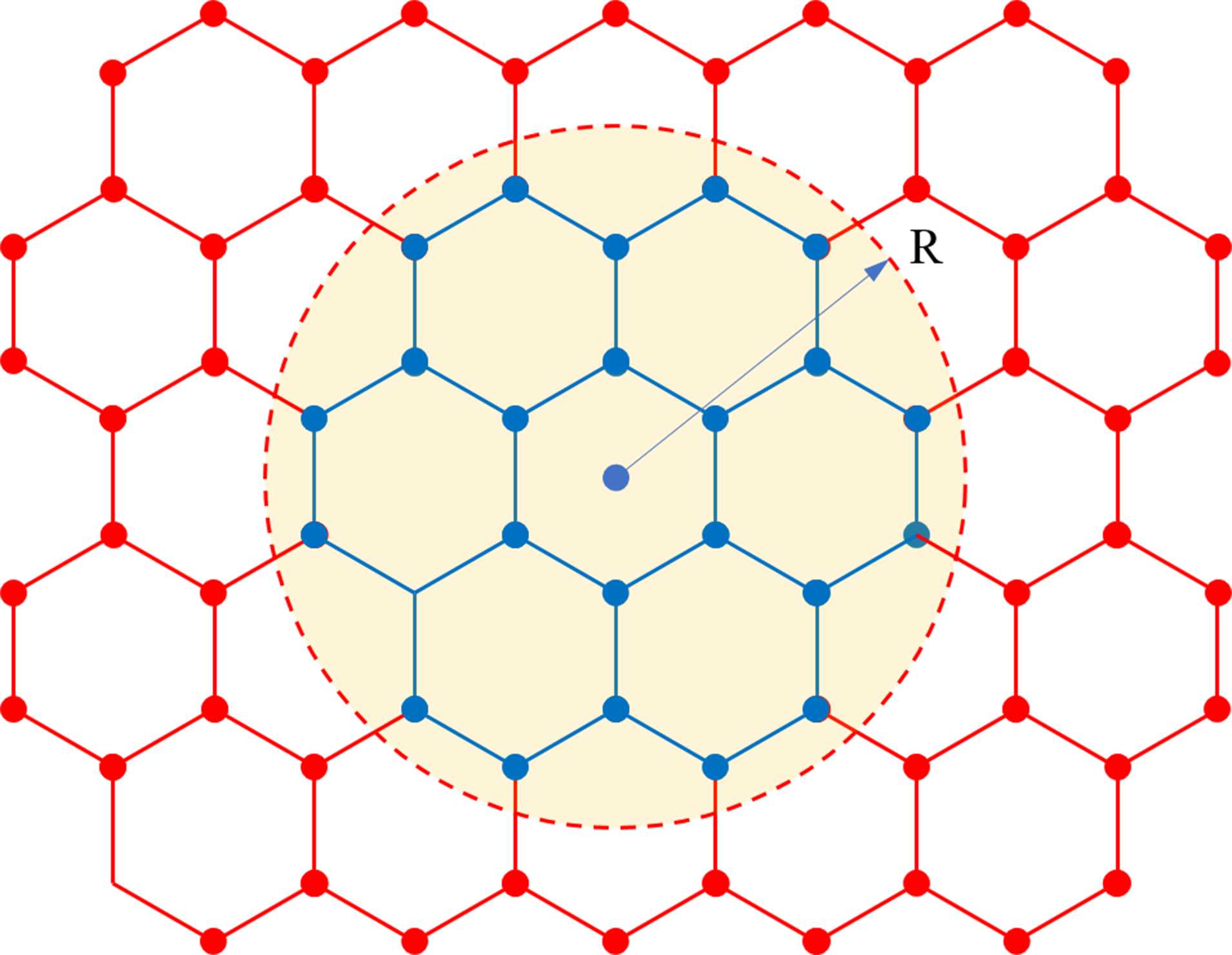
Topology optimization is gaining popularity as a primary tool for engineers in the initial stages of design. Essentially, the design domain is broken down into individual pixels, with the material density of each element or mesh point serving as a design variable. The optimization problem is then tackled through mathematical programming and optimization methods that rely on analytical gradient calculation. In this study, topology optimization using honeycomb tessellation elements is explored. Hexagonal elements have the ability to flexibly connect two adjacent elements. The use of the hexagonal element limits the occurrence of the checkerboard pattern to the finite elements of the quadrilateral standard Lagrangian type. A mathematical model is developed with the objective function being the minimum compliance value of the design domain. The element stiffness matrix is constructed using the strain-displacement matrix and the constitutive matrix, assuming a unit Young's modulus. Additionally, optimal conditions are established using Lagrangian multipliers. Two sensitivity and density filtering filters are employed to increase optimization efficiency, prevent the algorithm from reaching a local optimal state, and speed up convergence. If the suggested filter is employed, the objective function achieves a value of c=173,0293 and convergence is attained after 200 iterations. In contrast, without using the filter, the objective function has a larger value (c=186,7922) and convergence occurs at the 27th iteration. The results are significant for optimizing topology to meet specific boundary condition requirements. This paper proposes a novel approach using a combination of filters to advance topology optimization using hexagonal elements in future applications.

Author Biography

Ngoc-Tien Tran, Hanoi University of Industry

Doctor of Mechanical Engineering

Department of Mechatronics Engineering

References

  1. Sigmund, O. (2007). Morphology-based black and white filters for topology optimization. Structural and Multidisciplinary Optimization, 33 (4-5), 401–424. doi: https://doi.org/10.1007/s00158-006-0087-x
  2. Banh, T. T., Luu, N. G., Lee, D. (2021). A non-homogeneous multi-material topology optimization approach for functionally graded structures with cracks. Composite Structures, 273, 114230. doi: https://doi.org/10.1016/j.compstruct.2021.114230
  3. Huang, X., Li, W. (2022). Three-field floating projection topology optimization of continuum structures. Computer Methods in Applied Mechanics and Engineering, 399, 115444. doi: https://doi.org/10.1016/j.cma.2022.115444
  4. Zhou, P., Du, J., Lü, Z. (2018). A generalized DCT compression based density method for topology optimization of 2D and 3D continua. Computer Methods in Applied Mechanics and Engineering, 334, 1–21. doi: https://doi.org/10.1016/j.cma.2018.01.051
  5. Shen, W., Ohsaki, M. (2020). Geometry and topology optimization of plane frames for compliance minimization using force density method for geometry model. Engineering with Computers. doi: https://doi.org/10.1007/s00366-019-00923-w
  6. Tarek, M., Ray, T. (2020). Adaptive continuation solid isotropic material with penalization for volume constrained compliance minimization. Computer Methods in Applied Mechanics and Engineering, 363, 112880. doi: https://doi.org/10.1016/j.cma.2020.112880
  7. Mathai, B., Dhara, S., Gupta, S. (2022). Bone remodelling in implanted proximal femur using topology optimization and parameterized cellular model. Journal of the Mechanical Behavior of Biomedical Materials, 125, 104903. doi: https://doi.org/10.1016/j.jmbbm.2021.104903
  8. Li, H., Li, H., Gao, L., Li, J., Li, P., Yang, Y. (2021). Topology optimization of arbitrary-shape multi-phase structure with structured meshes based on a virtual phase method. Computer Methods in Applied Mechanics and Engineering, 387, 114138. doi: https://doi.org/10.1016/j.cma.2021.114138
  9. Liu, J., Zheng, Y., Ma, Y., Qureshi, A., Ahmad, R. (2019). A Topology Optimization Method for Hybrid Subtractive–Additive Remanufacturing. International Journal of Precision Engineering and Manufacturing-Green Technology, 7 (5), 939–953. doi: https://doi.org/10.1007/s40684-019-00075-8
  10. Sha, L., Lin, A., Zhao, X., Kuang, S. (2020). A topology optimization method of robot lightweight design based on the finite element model of assembly and its applications. Science Progress, 103 (3), 003685042093648. doi: https://doi.org/10.1177/0036850420936482
  11. Sato, A., Yamada, T., Izui, K., Nishiwaki, S., Takata, S. (2019). A topology optimization method in rarefied gas flow problems using the Boltzmann equation. Journal of Computational Physics, 395, 60–84. doi: https://doi.org/10.1016/j.jcp.2019.06.022
  12. Liu, S., Li, Q., Liu, J., Chen, W., Zhang, Y. (2018). A Realization Method for Transforming a Topology Optimization Design into Additive Manufacturing Structures. Engineering, 4 (2), 277–285. doi: https://doi.org/10.1016/j.eng.2017.09.002
  13. Xia, L., Zhang, L., Xia, Q., Shi, T. (2018). Stress-based topology optimization using bi-directional evolutionary structural optimization method. Computer Methods in Applied Mechanics and Engineering, 333, 356–370. doi: https://doi.org/10.1016/j.cma.2018.01.035
  14. Abdi, M., Ashcroft, I., Wildman, R. (2018). Topology optimization of geometrically nonlinear structures using an evolutionary optimization method. Engineering Optimization, 50 (11), 1850–1870. doi: https://doi.org/10.1080/0305215x.2017.1418864
  15. Hu, J., Yao, S., Gan, N., Xiong, Y., Chen, X. (2019). Fracture strength topology optimization of structural specific position using a bi-directional evolutionary structural optimization method. Engineering Optimization, 52 (4), 583–602. doi: https://doi.org/10.1080/0305215x.2019.1609466
  16. Rahmatalla, S. F., Swan, C. C. (2004). A Q4/Q4 continuum structural topology optimization implementation. Structural and Multidisciplinary Optimization, 27 (1-2), 130–135. doi: https://doi.org/10.1007/s00158-003-0365-9
  17. Talischi, C., Paulino, G. H., Pereira, A., Menezes, I. F. M. (2009). Polygonal finite elements for topology optimization: A unifying paradigm. International Journal for Numerical Methods in Engineering, 82 (6), 671–698. doi: https://doi.org/10.1002/nme.2763
  18. Kunakote, T., Bureerat, S. (2011). Multi-objective topology optimization using evolutionary algorithms. Engineering Optimization, 43 (5), 541–557. doi: https://doi.org/10.1080/0305215x.2010.502935
  19. Wallin, M., Ivarsson, N., Amir, O., Tortorelli, D. (2020). Consistent boundary conditions for PDE filter regularization in topology optimization. Structural and Multidisciplinary Optimization, 62 (3), 1299–1311. doi: https://doi.org/10.1007/s00158-020-02556-w
  20. Kumar, P., Saxena, A., Sauer, R. A. (2016). Implementation of Self Contact in Path Generating Compliant Mechanisms. Microactuators and Micromechanisms, 251–261. doi: https://doi.org/10.1007/978-3-319-45387-3_22
  21. Saxena, A. (2011). Topology design with negative masks using gradient search. Structural and Multidisciplinary Optimization, 44 (5), 629–649. doi: https://doi.org/10.1007/s00158-011-0649-4
  22. Sukumar, N., Tabarraei, A. (2004). Conforming polygonal finite elements. International Journal for Numerical Methods in Engineering, 61 (12), 2045–2066. doi: https://doi.org/10.1002/nme.1141
  23. Yoon, G. H., Ha, S. I. (2020). A New Development of a Shadow Density Filter for Manufacturing Constraint and Its Applications to Multiphysics Topology Optimization. Journal of Mechanical Design, 143 (6). doi: https://doi.org/10.1115/1.4048818
  24. Yi, B., Yoon, G. H., Peng, X. (2020). A simple density filter for the topology optimization of coated structures. Engineering Optimization, 53 (12), 2088–2107. doi: https://doi.org/10.1080/0305215x.2020.1845326
Topology optimization for isotropic elastic materials using honeycomb tessell

Downloads

Published

2023-04-30

How to Cite

Tran, N.-T. (2023). Topology optimization for isotropic elastic materials using honeycomb tessell. Eastern-European Journal of Enterprise Technologies, 2(7 (122), 43–49. https://doi.org/10.15587/1729-4061.2023.277909

Issue

Vol. 2 No. 7 (122) (2023): Applied mechanics

Section

Applied mechanics

License

Copyright (c) 2023 Ngoc-Tien Tran

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

The consolidation and conditions for the transfer of copyright (identification of authorship) is carried out in the License Agreement. In particular, the authors reserve the right to the authorship of their manuscript and transfer the first publication of this work to the journal under the terms of the Creative Commons CC BY license. At the same time, they have the right to conclude on their own additional agreements concerning the non-exclusive distribution of the work in the form in which it was published by this journal, but provided that the link to the first publication of the article in this journal is preserved.

A license agreement is a document in which the author warrants that he/she owns all copyright for the work (manuscript, article, etc.).
The authors, signing the License Agreement with TECHNOLOGY CENTER PC, have all rights to the further use of their work, provided that they link to our edition in which the work was published.
According to the terms of the License Agreement, the Publisher TECHNOLOGY CENTER PC does not take away your copyrights and receives permission from the authors to use and dissemination of the publication through the world's scientific resources (own electronic resources, scientometric databases, repositories, libraries, etc.).
In the absence of a signed License Agreement or in the absence of this agreement of identifiers allowing to identify the identity of the author, the editors have no right to work with the manuscript.
It is important to remember that there is another type of agreement between authors and publishers – when copyright is transferred from the authors to the publisher. In this case, the authors lose ownership of their work and may not use it in any way.