Оцінка можливості використання полімерної ракети-носія змінної довжини для виведення корисного навантаження

Автор(и)

  • Олександр Вячеславович Голубек Дніпровський національний університет імені Олеся Гончара, Україна https://orcid.org/0000-0002-7764-6278
  • Сергій Вікторович Алексєєнко Національний технічний університет «Дніпровська політехніка», Україна https://orcid.org/0000-0003-0320-989X
  • Микола Михайлович Дронь Дніпровський національний університет імені Олеся Гончара, Україна https://orcid.org/0000-0003-0682-8004
  • Андрій Юлійович Дреус Дніпровський національний університет імені Олеся Гончара, Україна https://orcid.org/0000-0003-0598-9287

DOI:

https://doi.org/10.15587/1729-4061.2024.306225

Ключові слова:

ракета-носій, змінна довжина, полімерний корпус, аеродинамічні характеристики, суборбітальна траєкторія

Анотація

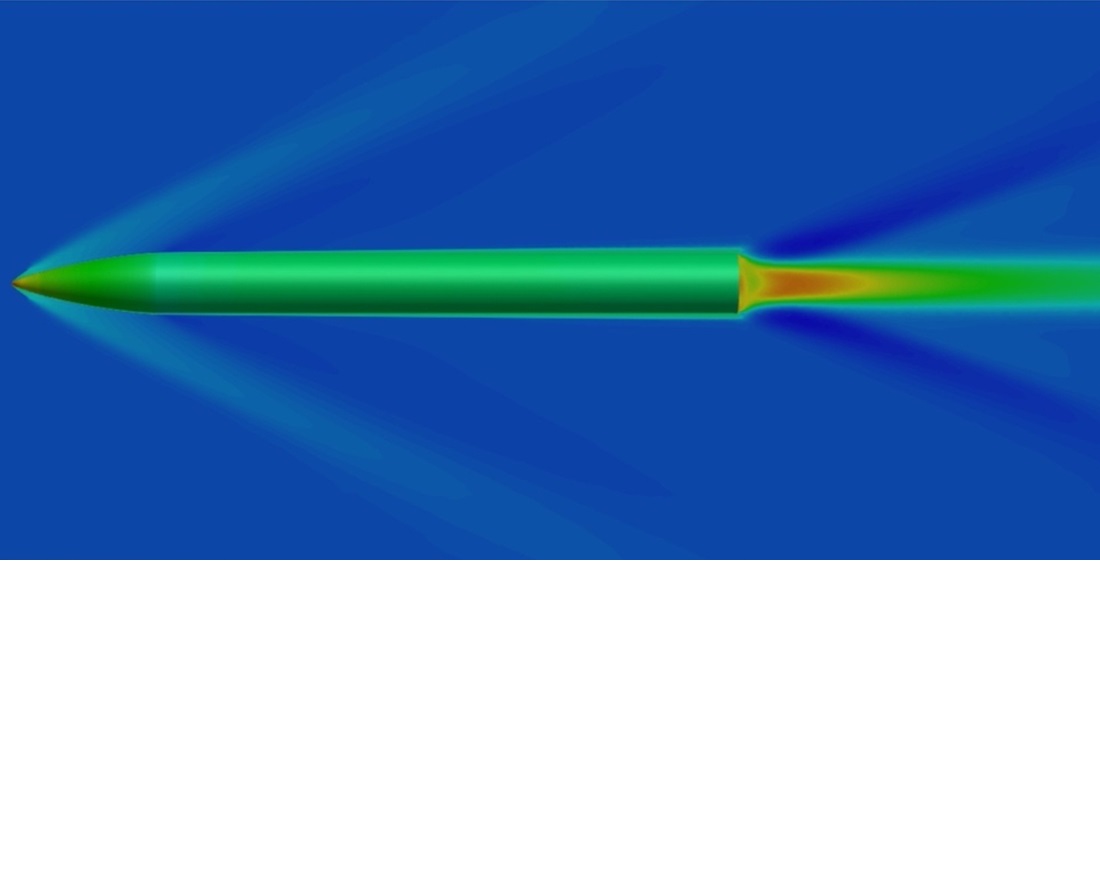
Об’єктом дослідження був рух ракети-носія надлегкого класу змінної довжини з полімерного корпусу на активній ділянці траєкторії. Робота присвячена вирішенню проблеми створення дешевих засобів доставлення на орбіту, а саме оцінці можливості виведення корисного навантаження ракетою-носієм з полімерним корпусом змінної довжини за межі щільної атмосфери Землі. Для цього виконано балістичне проєктування траєкторії ракети-носія з урахуванням перевантаження, визначено її аеродинамічні характеристики та особливості аеротермодинамічних процесів, що відбуваються під час польоту на атмосферній ділянці траєкторії. Показано близькість (до 10%) отриманих результатів з відомими експериментальними даними. Досліджено вплив сили аеродинамічного опору атмосфери Землі на параметри руху ракети-носія. Проведено моделювання польоту, результати якого показали принципову можливість виведення корисного навантаження класу CubeSat 24U за допомогою ракети-носія з полімерним корпусом змінної довжини  на суборбітальну траєкторію висотою порядку 300 км. При цьому діюче поздовжнє перевантаження на корпус ракети-носія не перевищує 4 одиниці, а температура на поверхні корпусу її ступені не перевищує 300°К. Особливістю проведеного дослідження є застосування мультидисциплінарного підходу, що визначається урахуванням взаємного зв’язку аеродинамічних, термодинамічних і балістичних процесів. Отримані параметри руху, аеродинамічні характеристики та температура нагріву поверхні корпусу ракети-носія є ключовими величинами для подальших досліджень щодо проєктування та аналізу ракети-носія з полімерним корпусом змінної довжини. Ці дані можуть бути використані для розрахунку механічних та теплових навантажень, що діють на конструкцію ракети-носія під час польоту

Біографії авторів

Олександр Вячеславович Голубек, Дніпровський національний університет імені Олеся Гончара

Доктор технічних наук, професор

Кафедра кібербезпеки та комп’ютерно-інтегрованих технологій

Сергій Вікторович Алексєєнко, Національний технічний університет «Дніпровська політехніка»

Доктор технічних наук, професор

Кафедра технологій машинобудування та матеріалознавства

Микола Михайлович Дронь, Дніпровський національний університет імені Олеся Гончара

Доктор технічних наук, професор

Кафедра ракетно-космічних та інноваційних технологій

Андрій Юлійович Дреус, Дніпровський національний університет імені Олеся Гончара

Доктор технічних наук, професор

Кафедра аерогідромеханіки та енергомасопереносу

Посилання

  1. Yemets, V., Sanin, F., Dzhur, Y., Masliany, M., Kostritsyn, O., Minteev, G. (2009). Single-stage small satellite launcher with combustible tank of polyethylene. Acta Astronautica, 64 (1), 28–32. https://doi.org/10.1016/j.actaastro.2008.06.015
  2. Yemets, V., Sanin, F., Masliany, M., Kostritsyn, O., Minteev, G. (2010). Is the combustible inertial pico launch vehicle feasible? JBIS - Journal of the British Interplanetary Society, 63 (7), 249–259.
  3. Yemets, V., Harkness, P., Dron’, M., Pashkov, A., Worrall, K., Middleton, M. (2018). Autophage Engines: Toward a Throttleable Solid Motor. Journal of Spacecraft and Rockets, 55 (4), 984–992. https://doi.org/10.2514/1.a34153
  4. Dron, M. M., Dubovyk, L. H., Holubek, O. V., Dreus, A. Yu., Yemets, A. V., Pashkov, A. V. (2019). Systemy vidvodu kosmichnykh obiektiv z nyzkykh navkolozemnykh orbit. Dnipro: LIRA, 218.
  5. Yemets, V., Dron, M., Pashkov, A., Dreus, A., Kositsyna, Ye., Yemets, M. et al. (2020). Method to preset G-load profile of launch vehicles. Proceedings of the International Astronautical Congress, IAC.
  6. Yemets, V., Dron’, M., Pashkov, A. (2020). Autophage Engines: Method to Preset Gravity Load of Solid Rockets. Journal of Spacecraft and Rockets, 57 (2), 309–318. https://doi.org/10.2514/1.a34597
  7. Yemets, V., Dron, M., Dreus, A., Pashkov, A., Yemets, M. (2021). Heat flows in the gasification chamber of the polymer propelled autophage launch vehicle. Proceedings of the International Astronautical Congress.
  8. Dreus, A., Yemets, V., Dron, M., Yemets, M., Golubek, A. (2021). A simulation of the thermal environment of a plastic body of a new type of launch vehicle at the atmospheric phase of the trajectory. Aircraft Engineering and Aerospace Technology, 94 (4), 505–514. https://doi.org/10.1108/aeat-04-2021-0100
  9. Dreus, А. Yu., Dron, М. М., Dubovik, L. G., Strembovsky, V. V. (2023). Assessment of the possibility of using polymers in the bodies of promising launch vehicles based on the heat resistance factor. Space Science and Technology, 29 (6), 03–12. https://doi.org/10.15407/knit2023.06.003
  10. Kondratiev, A. V. (2020). A concept of optimization of structural and technological parameters of polymer composite rocket units considering the character of their production. Space Science and Technology, 26 (6), 5–22. https://doi.org/10.15407/knit2020.06.005
  11. Kondratiev, A. V., Kovalenko, V. O. (2019). Optimization of design parameters of the main composite fairing of the launch vehicle under simultaneous force and thermal loading. Science and Technology, 25 (4), 3–21. https://doi.org/10.15407/knit2019.04.003
  12. Kondratiev, A., Potapov, O., Tsaritsynskyi, A., Nabokina, T. (2021). Optimal Design of Composite Shelled Sandwich Structures with a Honeycomb Filler. Advances in Design, Simulation and Manufacturing IV, 546–555. https://doi.org/10.1007/978-3-030-77719-7_54
  13. Kositsyna, O. S., Dron’, M. M., Yemets, V. V. (2020). The environmental impact assessment of emission from space launches: the promising propellants components selection. Journal of Chemistry and Technologies, 28 (2), 186–193. https://doi.org/10.15421/082020
  14. Kositsyna, O., Varlan, K., Dron, M., Kulyk, O. (2021). Determining energetic characteristics and selecting environmentally friendly components for solid rocket propellants at the early stages of design. Eastern-European Journal of Enterprise Technologies, 6 (6 (114)), 6–14. https://doi.org/10.15587/1729-4061.2021.247233
  15. Wang, L., He, G. Y., Wang, Q., Chen, L. S. (2020). An Engineering Method for Computing the Aerodynamics Performance of Hypersonic Vehicle. IOP Conference Series: Materials Science and Engineering, 816 (1), 012006. https://doi.org/10.1088/1757-899x/816/1/012006
  16. Yang, Z., Wang, S., Gao, Z. (2022). Studies on effects of wall temperature variation on heat transfer in hypersonic laminar boundary layer. International Journal of Heat and Mass Transfer, 190, 122790. https://doi.org/10.1016/j.ijheatmasstransfer.2022.122790
  17. Zhao, M. (2021). Prediction and Validation Technologies of Aerodynamic Force and Heat for Hypersonic Vehicle Design. In Springer Aerospace Technology. Springer Singapore. https://doi.org/10.1007/978-981-33-6526-1
  18. Dillenius, M. F. E., Nielsen, J. N. (1979). Computer Programs for Calculating Pressure Distributions Including Vortex Effects on Supersonic Monoplane or Cruciform Wing-Body-Tail Combinations with Round or Elliptical Bodies. NASA. Available at: https://ntrs.nasa.gov/citations/19810018506
  19. Cengizci, S., Uğur, Ö., Takizawa, K., Tezduyar, T. (2020). A Streamline-Upwind/Petrov-Galerkin Formulation For Supersonic and Hypersonic Flow Simulations. The 20th Biennial Computational Techniques and Applications Conference (CTAC2020). Available at: http://hdl.handle.net/11511/94032
  20. Li, J., Jiang, D., Geng, X., Chen, J. (2021). Kinetic comparative study on aerodynamic characteristics of hypersonic reentry vehicle from near-continuous flow to free molecular flow. Advances in Aerodynamics, 3 (1). https://doi.org/10.1186/s42774-021-00063-0
  21. Huang, T., He, G., Wang, Q. (2022). Calculation of Aerodynamic Characteristics of Hypersonic Vehicles Based on the Surface Element Method. Advances in Aerospace Science and Technology, 07 (02), 112–122. https://doi.org/10.4236/aast.2022.72007
  22. Furudate, M. A. (2022). MC-New: A Program to Calculate Newtonian Aerodynamic Coefficients Based on Monte-Carlo Integration. Aerospace, 9 (6), 330. https://doi.org/10.3390/aerospace9060330
  23. Thomas, P. D., Vinokur, M., Bastianon, R. A., Conti, R. J. (1972). Numerical Solution for Three-Dimensional Inviscid Supersonic Flow. AIAA Journal, 10 (7), 887–894. https://doi.org/10.2514/3.50241
  24. Hussein, A. K, Khan, W. A., Sivasankaran, S., Mohammed, H. A., Adegun, I. K. (2013). Numerical simulation of three-dimensional supersonic flow around an aerodynamic bump. Journal of Basic and Applied Scientific Research, 3 (7), 656–666.
  25. Marchenay, Y., Olazabal Loumé, M., Chedevergne, F. (2022). Hypersonic Turbulent Flow Reynolds-Averaged Navier–Stokes Simulations with Roughness and Blowing Effects. Journal of Spacecraft and Rockets, 59 (5), 1686–1696. https://doi.org/10.2514/1.a35339
  26. Sofair, I. (1995). Improved Method for Calculating Exact Geodetic Latitude and Altitude. Defense Technical Information Center. https://doi.org/10.21236/ada294568
  27. Bin, Y., Huang, G., Kunz, R., Yang, X. I. A. (2024). Constrained Recalibration of Reynolds-Averaged Navier–Stokes Models. AIAA Journal, 62 (4), 1434–1446. https://doi.org/10.2514/1.j063407
  28. Prihod'ko, A. A. (2008). Komp'yuternye tehnologii v aerogidrodinamike i teplomassoobmene. Kyiv: NAUKOVA DUMKA, 380.
  29. Prykhodko, O. A., Alekseyenko, S. V. (2014). Numerical simulation of the process of airfoil icing in the presence of large supercooled water drops. Technical Physics Letters, 40 (10), 864–867. https://doi.org/10.1134/s1063785014100125
  30. Prikhod’ko, A. A., Alekseenko, S. V. (2014). Numerical Simulation of the Processes of Icing on Airfoils with Formation of a “Barrier” Ice. Journal of Engineering Physics and Thermophysics, 87 (3), 598–607. https://doi.org/10.1007/s10891-014-1050-0
  31. Alekseyenko, S., Dreus, A., Dron, M., Brazaluk, O. (2022). Numerical Study of Aerodynamic Characteristics of a Pointed Plate of Variable Elongation in Subsonic and Supersonic Gas Flow. Journal of Advanced Research in Fluid Mechanics and Thermal Sciences, 96 (2), 88–97. https://doi.org/10.37934/arfmts.96.2.8897
  32. Gutiérrez, R., Llorente, E., Ragni, D., Aranguren, P. (2022). Study on k−ω−Shear Stress Transport Corrections Applied to Airfoil Leading-Edge Roughness Under RANS Framework. Journal of Fluids Engineering, 144 (4). https://doi.org/10.1115/1.4052925
  33. Adanta, D., Fattah, I. M. R., Muhammad, N. M. (2020). Comparison of standard k-epsilon and SST k-omega turbulence model for breastshot waterwheel simulation. Journal of Mechanical Science and Engineering, 7 (2), 039–044. https://doi.org/10.36706/jmse.v7i2.44
  34. Costa, L. M. F., Montiel, J. E. S., Corrêa, L., Lofrano, F. C., Nakao, O. S., Kurokawa, F. A. (2022). Influence of standard k-ε, SST κ-ω and LES turbulence models on the numerical assessment of a suspension bridge deck aerodynamic behavior. Journal of the Brazilian Society of Mechanical Sciences and Engineering, 44 (8). https://doi.org/10.1007/s40430-022-03653-1
  35. Maurício Araújo, A., Antônio Coutinho Silva, A., Leal dos Santos, C., Corte Real Fernandes, E., Menezes, E., José Arruda Moura Rocha, G. et al. (2017). An assessment of different turbulence models on a CFD simulation of air flow past a S814 airfoil. Procceedings of the 24th ABCM International Congress of Mechanicl Engineering. https://doi.org/10.26678/abcm.cobem2017.cob17-0306
  36. Robinson, D., Hassan, H. (1996). A two-equation turbulence closure model for wall bounded and free shear flows. Fluid Dynamics Conference. https://doi.org/10.2514/6.1996-2057
  37. Petrov, K. P. (1998). Aerodinamika tel prosteyshih form. Moscow: Fizmatlit, 428.
  38. Meng, Y., Yan, L., Huang, W., Tong, X. (2020). Numerical Investigation of the Aerodynamic Characteristics of a Missile. IOP Conference Series: Materials Science and Engineering, 887 (1), 012001. https://doi.org/10.1088/1757-899x/887/1/012001
  39. Bayley, D. J., Hartfield, R. J., Burkhalter, J. E., Jenkins, R. M. (2008). Design Optimization of a Space Launch Vehicle Using a Genetic Algorithm. Journal of Spacecraft and Rockets, 45 (4), 733–740. https://doi.org/10.2514/1.35318
Оцінка можливості використання полімерної ракети-носія змінної довжини для виведення корисного навантаження

##submission.downloads##

Опубліковано

2024-06-28

Як цитувати

Голубек, О. В., Алексєєнко, С. В., Дронь, М. М., & Дреус, А. Ю. (2024). Оцінка можливості використання полімерної ракети-носія змінної довжини для виведення корисного навантаження. Eastern-European Journal of Enterprise Technologies, 3(7 (129), 63–72. https://doi.org/10.15587/1729-4061.2024.306225

Номер

Том 3 № 7 (129) (2024): Прикладна механіка

Розділ

Прикладна механіка

Ліцензія

Авторське право (c) 2024 Aleksandr Golubek, Serhii Aleksieienko, Mykola Dron, Andrii Dreus

Creative Commons License

Ця робота ліцензується відповідно до Creative Commons Attribution 4.0 International License.

Закріплення та умови передачі авторських прав (ідентифікація авторства) здійснюється у Ліцензійному договорі. Зокрема, автори залишають за собою право на авторство свого рукопису та передають журналу право першої публікації цієї роботи на умовах ліцензії Creative Commons CC BY. При цьому вони мають право укладати самостійно додаткові угоди, що стосуються неексклюзивного поширення роботи у тому вигляді, в якому вона була опублікована цим журналом, але за умови збереження посилання на першу публікацію статті в цьому журналі.

Ліцензійний договір – це документ, в якому автор гарантує, що володіє усіма авторськими правами на твір (рукопис, статтю, тощо).
Автори, підписуючи Ліцензійний договір з ПП «ТЕХНОЛОГІЧНИЙ ЦЕНТР», мають усі права на подальше використання свого твору за умови посилання на наше видання, в якому твір опублікований. Відповідно до умов Ліцензійного договору, Видавець ПП «ТЕХНОЛОГІЧНИЙ ЦЕНТР» не забирає ваші авторські права та отримує від авторів дозвіл на використання та розповсюдження публікації через світові наукові ресурси (власні електронні ресурси, наукометричні бази даних, репозитарії, бібліотеки тощо).
За відсутності підписаного Ліцензійного договору або за відсутністю вказаних в цьому договорі ідентифікаторів, що дають змогу ідентифікувати особу автора, редакція не має права працювати з рукописом.
Важливо пам’ятати, що існує і інший тип угоди між авторами та видавцями – коли авторські права передаються від авторів до видавця. В такому разі автори втрачають права власності на свій твір та не можуть його використовувати в будь-який спосіб.